Exact Differential Equation

Let's begin by understanding what differential equations are. A differential equation is an equation involving one or more terms and the derivatives of one dependent variable with respect to the other independent variable. An exact differential equation is a specific type of ordinary differential equation commonly utilized in physics and engineering. Exact differential equations are widely used in thermodynamics for calculating internal energy and also used in electrostatics for calculating electric potential.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

In this article, we will cover the Exact differential equations. This concept falls under the broader category of differential equations. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of five questions have been asked on this concept, including one in 2019, one in 2020, two in 2021, and one in 2022.

What is a Differential Equation?

A differential equation is an equation involving one or more terms and the derivatives of one dependent variable with respect to the other independent variable.

Differential equation: $\frac{d y}{d x}=f(x)$
Where " $x$ " is an independent variable and " $y$ " is a dependent variable

Example of differential equation: $x \frac{d y}{d x}+2 y=0$
The above-written equation involves variables as well as the derivative of the dependent variable $\mathrm{y}$ with respect to the independent variable $\mathrm{x}$. Therefore, it is a differential equation.
The following relations are some of the examples of differential equations:
(i) $\frac{d y}{d x}=\sin 2 x+\cos x$
(ii) $\mathrm{k} \frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}=\left[1+\left(\frac{\mathrm{dy}}{\mathrm{dx}}\right)^2\right]^{3 / 2}$

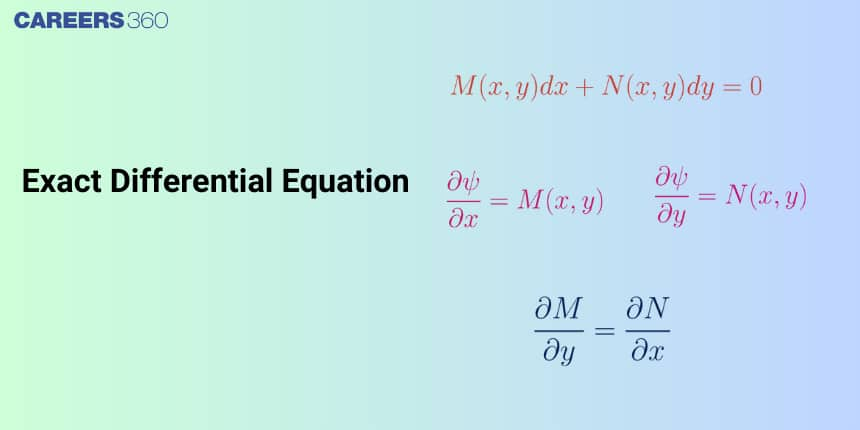
Exact Differential Equation

The equation $\mathrm{A}(\mathrm{x}, \mathrm{y}) \mathrm{dx}+\mathrm{B}(\mathrm{x}, \mathrm{y}) \mathrm{dy}=0$ is an exact differential equation if there exists a function of two variables $x$ and $y$ having continuous partial derivatives such that the exact differential equation definition is separated as follows

$
u_x(x, y)=A(x, y) \text { and } u_y(x, y)=B(x, y)
$

General Form

The general form of the exact differential equation is $A(x, y) d x+B(x, y) d y=0$ where A and B are the polynomial functions in terms of x and y.

Sometimes some differential equations can be solved using observation only. In such equations, we can get a differential of a function of x and y.

The equation $A(x, y) d x+B(x, y) d y=0$ is an exact differential equation if there exists a function of two variables $x$ and $y$ having continuous partial derivatives such that the exact differential equation definition is separated as follows $u_x(x, y)=A(x, y)$ and $u_y(x, y)=B(x, y)$

General Form
Sometimes some differential equations can be solved using observation only. In such equations, we can get a differential of a function of x and y .

Exact Differential Equation Integrating Factor

If the differential equation $A(x, y) d x+B(x, y) d y=0$ is not exact, it is possible to make it exact by multiplying using a relevant factor $\mathrm{u}(\mathrm{x}, \mathrm{y})$ which is known as integrating factor for the given differential equation.

Consider an example,

$
2 y d x+x d y=0
$

Now check it whether the given differential equation is exact using testing for exactness.
The given differential equation is not exact.
In order to convert it into the exact differential equation, multiply by the integrating factor $u(x, y)=x$, the differential equation becomes,

$
2 x y d x+x^2 d y=0
$

The above resultant equation is an exact differential equation because the left side of the equation is a total differential of $x^2 y$.

Sometimes it is difficult to find the integrating factor. But, there are two classes of differential equations whose integrating factor may be found easily. Those equations have the integrating factor having the functions of either $x$ alone or $y$ alone.

When you consider the differential equation $A(x, y) d x+B(x, y) d y=0$, the two cases involved are:

Case 1: If $[1 / B(x, y)]\left[A_y(x, y)-B_x(x, y)\right]=h(x)$, which is a function of $x$ alone, then $e^{\int h(x) d x}$ is an integrating factor.

Case 2: If $[1 / A(x, y)]\left[B_x(x, y)-A_y(x, y)\right]=k(y)$, which is a function of $y$ alone, then $e^{j k(y) d y}$ is an integrating factor.

How to Solve Exact Differential Equations

The following steps explain how to solve the exact differential equation:

Step 1: The first step to solving the exact differential equation is to make sure the given differential equation is exact.

$
\frac{\partial Q}{\partial x}=\frac{\partial P}{\partial y}
$

Step 2: Write the system of two differential equations that defines the function $u(x, y)$. That is

$
\begin{aligned}
& \frac{\partial u}{\partial x}=P(x, y) \\
& \frac{\partial u}{\partial y}=Q(x, y)
\end{aligned}
$

Step 3: Integrating the first equation over the variable $x$, we get

$
u(x, y)=\int P(x, y) d x+\phi(y)
$

Step 4: Differentiating concerning $y$, substitute the function $u(x, y)$ in the second equation

$
\frac{\partial u}{\partial x}=\frac{\partial}{\partial x}\left[\int P(x, y) d x+\phi(y)\right]=Q(x, y)
$

Step 5: We can find the function $\varphi(y)$ by integrating the last expression so that the function $\mathrm{u}(\mathrm{x}, \mathrm{y})$ becomes

$
u(x, y)=\int P(x, y) d x+\phi(y)
$

Step 6: Finally, the general solution of the exact differential equation is given by

$
u(x, y)=C
$
Illustration 1 :
Solution of the differential equation $2 x y d x+\left(x^2+3 y^2\right) d y=0$ is Let us first separate terms containing only x with dx and terms containing only y with dy

$
2 x y d x+x^2 d y+3 y^2 d y=0
$

Here first two terms have both $x$ and $y$. We can make an observation that first two terms are the differentiation of $x^2 y$. Hence we can write this equation as

$
d\left(x^2 y\right)+3 y^2 d y=0
$

Integrating this, we get

$
x^2 y+y^3+c=0
$
This is the solution of this equation

The presence of the following exact differentials should be observed in a given differential equation

1. $x d y+y d x=d(x y)$
2. $x d x+y d y=\frac{1}{2} d\left(x^2+y^2\right)$
3. $\frac{x d y-y d x}{x^2}=d\left(\frac{y}{x}\right)$
4. $\frac{y d x-x d y}{y^2}=d\left(\frac{x}{y}\right)$
5. $\frac{x d y-y d x}{x y}=\frac{d y}{y}-\frac{d x}{x}=d\left[\log \left(\frac{y}{x}\right)\right]$
6. $\frac{y d x-x d y}{x y}=d\left[\log \left(\frac{x}{y}\right)\right]$

Illustration 2:

$
\frac{x d y-y d x}{x^2+y^2}+e^x d x=0
$

Observe that

$
\frac{x d y-y d x}{x^2+y^2}=\frac{\frac{x d y-y d x}{x^2}}{1+\frac{y^2}{x^2}}=\frac{d\left(\frac{y}{x}\right)}{1+\left(\frac{y}{x}\right)^2}=d\left[\tan ^{-1}\left(\frac{y}{x}\right)\right]
$

So the equation is

$
d\left[\tan ^{-1} \frac{y}{x}\right]+e^x d x=0
$
Integrating

$\tan ^{-1} \frac{y}{x}+e^x+c=0$

Recommended Video Based on Exact Differential Equations

Solved Examples Based On Exact Differential Equations

Example 1: The solution of the differential equation dy/dx = -1 is

Solution:

$
\begin{aligned}
& d(x+y)=d x+d y \\
& \frac{d y}{d x}=-1 \Rightarrow d x+d y=0 \Rightarrow d(x+y)=0 \\
& \Rightarrow \int d(x+y)=C \Rightarrow(x+y)=C
\end{aligned}
$

Hence, the answer is $\mathrm{x}+\mathrm{y}=\mathrm{C}$.
Example 2: The solution of a differential equation $\frac{d y}{d x}=\frac{-\cos x}{\cos y}$ is
Solution:
As we have learned
The general form of Variable Separation -

$
\begin{aligned}
& d(x+y)=d x+d y \\
& \cos x d x+\cos y d y=0 \text { is also a linear differential eq } \\
& d \sin x+d(\sin y)=0 \Rightarrow d(\sin x+\sin y)=0 \\
& \int d(\sin x+\sin y)=C \Rightarrow \sin x+\sin y=C
\end{aligned}
$

$
\cos x d x+\cos y d y=0 \text { is also a linear differential equation that we can write }
$

Hence, the answer is $\sin x+\sin y=C$..

Example 3: Solution of differential equation $\sin x \cos y d y+\sin y \cos x d x$
Solution:
As we have learned
The general form of Variable Separation -

$
d(x y)=y d x+x d y
$

The equation can be written as

$
\begin{aligned}
& (\sin x) d(\sin y)+(\sin y) d(\sin x)=0 \Rightarrow d(\sin x \cdot \sin y)=0 \\
& \Rightarrow \int d(\sin x \cdot \sin y)=C \Rightarrow \sin x \cdot \sin y=C
\end{aligned}
$

Hence, the answer is $\sin x \cdot \sin y=C$.

Example 4: The solution of the differential equation $(2 x y-\sin x) d x+\left(\left(x^2\right)-\cos y\right) d y=0$ is

Solution:
As we have learned

The general form of Variable Separation -

$
d(x y)=y d x+x d y
$

The equation can be written as

$
\begin{aligned}
& 2 x y d x-\sin x d x+x^2 d y-\cos y d y=0 \\
& 2 x y d x+x^2 d y-\sin x d x-\cos y d y=0 \\
& \Rightarrow y(2 x) d x+x^2 d y-\sin x d x-\cos y d y=0 \\
& \Rightarrow d\left(y \cdot x^2\right)+d(\cos x)-d(\sin y)=0 \\
& \Rightarrow \int d\left(y \cdot x^2\right)+\int d(\cos x)-\int d(\sin y)=C \\
& \Rightarrow x^2 y+\cos x-\sin y=C
\end{aligned}
$

Hence, the answer is $x^2 y+\cos x-\sin y=C$

Example 5: The solution of the differential equation $y d x / d y=x+2 \sqrt{ } y^2-x^2$ is
Solution:
As we have learned
The general form of Variable Separation -

$
d\left(\frac{x}{y}\right)=\frac{y d x-x d y}{y^2}
$

The equation can be written as

$
\begin{aligned}
& y d x=x d y+2 \sqrt{y^2-x^2} d y \\
& \Rightarrow y d x-x d y=2 \sqrt{y^2-x^2} d y \\
& \Rightarrow y d x-x d y=2 y \sqrt{1-x^2 / y^2} d y
\end{aligned}
$

dividing both sides by $y^2$ we get

$
\begin{aligned}
& \frac{y d x-x d y}{y^2}=\frac{2 \sqrt{1-(x / y)^2}}{y} d y \\
& \Rightarrow \frac{d(x / y)}{\sqrt{1-(x / y)^2}}=2 / y d y
\end{aligned}
$

on integrating, we get

$
\sin ^{-1} x / y=2 \ln |y|+C
$
Hence, the answer is $\sin ^{-1} x / y=2 \ln |y|+C$ .

Summary

Exact differential equations are a powerful tool in mathematics and physics, providing a method for finding potential functions from differential relationships among them. These equations are expanded in various applications and fields, including thermodynamics, electrostatics, and fluid mechanics, where they help to describe fundamental physical processes with the help of integrable functions.

Frequently Asked Question(FAQ):

1. What is a differential equation?

Ans- It describes the rate of change in quantity and is used in science, engineering, business, etc. It can model many phenomena in different fields.

2. What is an exact differential equation?

Ans: An exact differential equation is a type of first-order differential equation that is solved with the help of integration.

3. What is the order of the exact differential equations?

Ans: Exact differential equations are generally first order.

4. How to solve exact differential equations?

Ans: Exact differential equations are generally solved by observations and after this, we integrate them.

5. Write some applications of exact differential equations.

Ans: Exact differential equations are widely used in thermodynamics, electrostatics, and fluid mechanics.