Formation of Differential Equation and Solutions of a Differential Equation

A differential equation is a mathematical tool or equation that relates or equates a polynomial types function with its derivatives. Formation of a differential equation involves different processes like deriving a differential equation representing a given family of functions or a specific real-life scenario. This type of technique helps in creating or analyzing various forces and understanding their behaviour through mathematical analysis.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. What is a Differential Equation?
2. Formation of Differential Equation
3. Solution of Differential Equations
4. Solved Examples Based On Formation of Differential Equations and its Solution
5. Summary

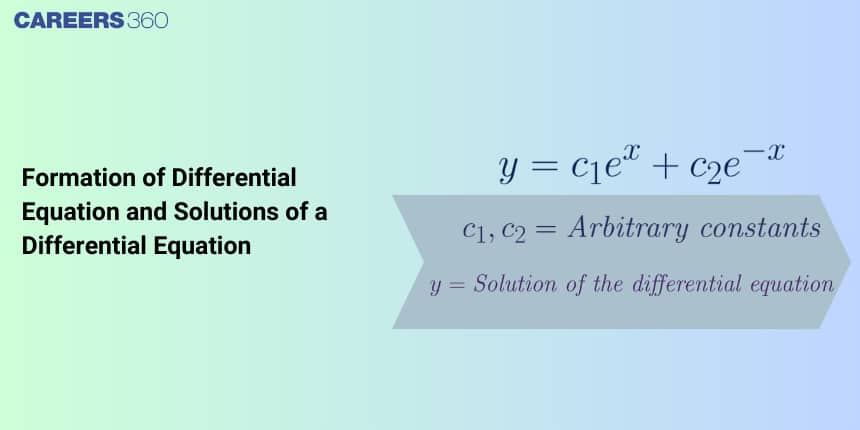
In this article, we will cover the concept of the formation of a Differential Equation and the Solutions of a Differential Equation. This concept falls under the broader category of differential equations, which is a crucial Chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of ten questions have been asked on this concept, including two in 2014, three in 2017, three in 2019, one in 2021, and one in 2023.

What is a Differential Equation?

A differential equation is an equation involving one or more terms and the derivatives of one dependent variable with respect to the other independent variable.

Differential equation: dy/dx = f(x)

Where “x” is an independent variable and “y” is a dependent variable

Example of differential equation: $x \frac{d y}{d x}+2 y=0$
The above-written equation involves variables as well as the derivative of the dependent variable $\mathrm{y}$ with respect to the independent variable $\mathrm{x}$. Therefore, it is a differential equation.
The following relations are some of the examples of differential equations:
(i) $\frac{d y}{d x}=\sin 2 x+\cos x$
(ii) $\mathrm{k} \frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}=\left[1+\left(\frac{\mathrm{dy}}{\mathrm{dx}}\right)^2\right]^{3 / 2}$

Formation of Differential Equation

We have studied the general formula of the parabola which is y² = 4ax

This equation represents a family of parabolas with an arbitrary constant. With different values of a, we get a different parabola in this family.

To form its D.E, let us first differentiate it w.r.t. x,

$
2 \mathrm{y} \frac{\mathrm{dy}}{\mathrm{dx}}=4 \mathrm{a} \quad \text { or } \quad \frac{\mathrm{y}}{2} \frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{a}
$

Putting this value of 'a' in the original equation of the parabola

$
\begin{aligned}
& y^2=4 \cdot \frac{y}{2} \frac{d y}{d x} \cdot x \\
& 2 x \frac{d y}{d x}=y
\end{aligned}
$
This is the Differential Equation for a family of parabolas y² = 4ax.

Note that there is one arbitrary constant in the original equation, and the order of the D.E. formed is also 1. This is an important result: if an equation contains n arbitrary constants, then its D.E. will have an order equal to n.

If we are given a relation between the variables x, y with n arbitrary constants C₁, C₂, .... C_n, then to form its D.E., we differentiate the given relation n times in succession with respect to x, we have n + 1 equations altogether. Now using these we eliminate the n arbitrary constants. The result is a differential equation of the nth order.

Consider the equation of the family of ellipses with a and b as arbitrary constants

This is the D.E. that represents the given family of ellipses

Consider the equation of the family of ellipses with $a$ and $b$ as arbitrary constants

$
\frac{\mathrm{x}^2}{\mathrm{a}^2}+\frac{\mathrm{y}^2}{\mathrm{~b}^2}=1
$

Differentiate Eq (4) w.r.t. 'x ${ }^{\prime}$

$
\frac{2 \mathrm{x}}{\mathrm{a}^2}+\frac{2 \mathrm{y}}{\mathrm{b}^2} \cdot\left(\frac{\mathrm{dy}}{\mathrm{dx}}\right)=0 \quad \text { or } \quad-\frac{\mathrm{b}^2}{\mathrm{a}^2}=\frac{\mathrm{yy}^{\prime}}{\mathrm{x}}
$

Differentiate above equation w.r.t. ' $x$ '

$
\begin{gathered}
0=\frac{\mathrm{x}\left(\mathrm{y}^{\prime 2}+\mathrm{yy}^{\prime \prime}\right)-\mathrm{yy}^{\prime}}{\mathrm{x}^2} \\
\mathrm{yy}^{\prime}=\mathrm{xyy}^{\prime \prime}+\mathrm{x}\left(\mathrm{y}^{\prime}\right)^2
\end{gathered}
$

Illustration :

Differential equation of the equation $y=(a+b) e^x+e^{x+c}$ is Here, the number of the arbitrary constant is $3: a, b$, and $c$. But we can club arbitrary constants together

$
y=\left(a+b+e^c\right) e^x
$

which is of the form, $\mathrm{y}=\mathrm{Ae}^{\mathrm{x}} \quad$ where, $\mathrm{A}=\left(\mathrm{a}+\mathrm{b}+\mathrm{e}^{\mathrm{c}}\right)$ hence, corresponding Differential equation will be of order 1

$
\begin{aligned}
& \Rightarrow \frac{d y}{d x}=A e^x \\
& \Rightarrow \frac{d y}{d x}=y
\end{aligned}
$

Solution of Differential Equations

Solutions can be classified according to different types of equations and given conditions. There are different types of solutions for differential equations based on their types or structure differential equations. General solutions and particular solutions are two types of differential equation solutions.

If the given D.E. is

$
\begin{aligned}
& 2 x \frac{d y}{d x}=y \\
& 2 \frac{d y}{y}=\frac{d x}{x} \\
& 2 \int \frac{d y}{y}=\int \frac{d x}{x} \\
& 2 \ln (y)=\ln (x)+c
\end{aligned}
$

As $c$ is a constant of integration, it can take any real value. We can write it as $\ln (4 a)$ as well, where a is now the arbitrary constant.

$
\begin{aligned}
& \ln \left(y^2\right)=\ln (x)+\ln (4 a) \\
& y^2=4 a x
\end{aligned}
$
This is the general solution of the differential equation, which represents the family of the parabola

If we put some real value of a in this equation, then we get a particular solution of the given differential equation. For example, if we put a = 2, then y² = 8x is a particular solution of this equation.

Hence, the solution of the differential equation is a relation between the variables of the equation that satisfy the D.E. and does not contain any derivatives or any arbitrary constants.

A general solution of a differential equation is a relation between the variables (not involving the derivatives) which contains the same number of the arbitrary constants as the order of the differential equation.

A particular solution of the differential equation is obtained from the general solution by assigning particular values to the arbitrary constant in the general solution.

To solve the first-order differential equation of first degree, some standard forms are available to get the general solution. They are:

• Variable separable method
• Reducible into the variable separable method
• Linear differential equation
• Reducible into a linear differential equation
• Exact differential equations
• Linear differential equations with constant coefficients

Note: If arbitrary constants appear in addition, subtraction, multiplication, or division, then we can club them to reduce into one new arbitrary constant. Hence, the differential equation corresponding to a family of curves will have the order the same as the number of essential arbitrary constants (number of arbitrary constants in the modified form) in the curve equation.

Recommended Video Based on Formation of Differential Equations and its Solution

Solved Examples Based On Formation of Differential Equations and its Solution

Example 1: The differential equation for the family of curves $x^2+y^2-2 a y=0$, where $a$ is an arbitrary constant is.
(1) $\left(x^2-y^2\right) y^{\prime}=2 x y$
(2) $2\left(x^2+y^2\right) y^{\prime}=x y$
(3) $2\left(x^2-y^2\right) y^{\prime}=x y$
(4) $\left(x^2+y^2\right) y^{\prime}=2 x y$

Solution:

$
\begin{aligned}
& x^2+y^2-2 a y=0 \\
& 2 x+2 y \frac{d y}{d x}-2 a \cdot \frac{d y}{d x}=0 \\
& \Rightarrow x+y \frac{d y}{d x}=a \frac{d y}{d x} \\
& \Rightarrow x+y \frac{d y}{d x}=\frac{x^2+y^2}{2 y} \cdot \frac{d y}{d x} \\
& \Rightarrow 2 x y+2 y^2 \frac{d y}{d x}=x^2 \frac{d y}{d x}+y^2 \frac{d y}{d x} \\
& \Rightarrow\left(x^2-y^2\right) \frac{d y}{d x}=2 x y \\
& \Rightarrow\left(x^2-y^2\right) y^{\prime}=2 x y
\end{aligned}
$
Hence, the answer is option (1).

Example 2:The differential equation which represents the family of curves $y=c_1 e^{c_2 x}$, where $c_1$ and $c_2$ are arbitrary constants, is
(1) $y^{\prime \prime}=y^{\prime} y$
(2) $y y^{\prime \prime}=y^{\prime}$
(3) $y y^{\prime \prime}=\left(y^{\prime}\right)^2$
(4) $y^{\prime}=y^2$

Solution: $\square$
As we learned in
Formation of Differential Equations -
A differential equation can be derived from its equation by the process of differentiation and another algebraical process of elimination

$
\begin{aligned}
& y=C_1 \cdot e^{C_2 x} \\
& y^{\prime}=C_1 \cdot e^{C_2 x} \times C_2 \Rightarrow C_1 C_2 \cdot e^{C_2 x}=C_2 y \\
& y^{\prime \prime}=C_2 \cdot y^{\prime} \\
& \Rightarrow \frac{y^{\prime}}{y^{\prime \prime}}=\frac{y}{y^{\prime}} \\
& \Rightarrow y y^{\prime \prime}=\left(y^{\prime}\right)^2
\end{aligned}
$

Hence, the answer is the option (3).

Example 3: If the differential equation representing the family of all circles touching the $x$ - axis at the origin is $\left(x^2-y^2\right) \frac{d y}{d x}=g(x) y$, then $g(x)$ equals :
(1) $\frac{1}{2} x$
(2) $2 x^2$
(3) $2 x$
(4) $\frac{1}{2} x^2$

Solution:
As we learned in
Formation of Differential Equations -
A differential equation can be derived from its equation by the process of differentiation and another algebraical process of elimination

$
\begin{aligned}
& \Rightarrow 2 x+2(y-a) \frac{d y}{d x}=0 \\
& \therefore x+(y-a) \frac{d y}{d x}=0 \\
& \therefore \frac{d y}{d x}=\frac{x}{(y-a)} \quad \text { or } \quad a=\frac{x+y \frac{d y}{d y}}{d y / d x}
\end{aligned}
$

Put a in (i)

$
\begin{aligned}
& x^2+\frac{x^2}{(d y / d x)^2}=\left(\frac{x+y \frac{d y}{d x}}{(d y / d x)}\right)^2 \\
& \Rightarrow\left(x^2-y^2\right) \frac{d y}{d x}=2 x y \\
& \therefore g(x)=2 x
\end{aligned}
$
Hence, the answer is option (3).

Example 4: The differential equation whose solution is $A x^2+B y^2=1$, where $A$ and $B$ are arbitrary constant, is of
(1) second-order and second-degree
(2) first-order and second degree
(3) first-order and first-degree
(4) second-order and first-degree

Solution:
After forming the differential equation, its order and degree can be determined.

$
A x^2+B y^2=1
$$

Differentiating, we get:

$
\begin{aligned}
& \Rightarrow 2 A x+2 B y \frac{d y}{d x}=0 \\
& \Rightarrow A x+B y \frac{d y}{d x}=0
\end{aligned}
$$

Differentiating, we get:

$
\begin{aligned}
& \Rightarrow A+B\left[\frac{d y}{d x} \cdot \frac{d y}{d x}+y \cdot \frac{d^2 y}{d x^2}\right]=0 \\
& \Rightarrow A+B\left[\left(\frac{d y}{d x}\right)^2+y \frac{d^2 y}{d x^2}\right]=0
\end{aligned}
$
By eliminating A and B, we get:

$x y y^{\prime \prime}+x\left(y^{\prime}\right)^2-y y^{\prime}=0$

Here, Order = 2 and Degree = 1.

Hence, the answer is option (4).

Summary

The formation of differential equations from a given set of conditions or family of functions involves differentiating and eliminating arbitrary constants. Solutions to differential equations provide functions that satisfy the equations and can be general, particular, or singular. Various methods, including separation of variables, integrating factors, and numerical techniques, are used to solve these equations, making differential equations powerful tools for modeling and analyzing dynamic systems.

Frequently Asked Questions(FAQs)

1. What is a differential equation?

Ans- It describes the rate of change in quantity and is used in science, engineering, business, etc. It can model many phenomena in different fields.

2. What is the process of forming a differential equation from a given family of functions?

Ans: The process involves:
(i) Identifying the family of functions that include arbitrary constants.
(ii) Differentiating the function with respect to the independent variable to obtain as many equations as there are arbitrary constants.
(iii) Eliminating the arbitrary constants to form a differential equation that represents the given family of functions.

3. How do you eliminate arbitrary constants to form a differential equation?

Ans: After differentiating the given family of functions, you use the original function and its derivatives to solve for the arbitrary constants. Substituting these expressions back into the differentiated equations allows you to eliminate the constants, resulting in a differential equation.

4. What is a general solution of a differential equation?

Ans: The general solution of a differential equation includes all possible solutions and contains arbitrary constants. For example, the general solution to $\frac{\mathrm{d} y}{\mathrm{~d} x}=k y$ is $y=C e^{k x}$ where C is an arbitrary constant.
5. What is a particular solution of a differential equation?

Ans: A particular solution is obtained by assigning specific values to the arbitrary constants in the general solution, often based on initial or boundary conditions. For example, if $Y(0)=y_0$ the particular solution to $\frac{\mathrm{d} y}{\mathrm{~d} x}=k y$ is $y=y_0 e^{k x}$