Fundamental Principle Of Counting

Fundamental Principle of Counting

Edited By Komal Miglani | Updated on Jul 02, 2025 05:35 PM IST

The fundamental counting principle is a rule used to determine the total number of possible outcomes in a given situation. This principle helps us in solving various problems related to permutations and combinations, enabling us to make informed choices from all available options. The fundamental principles of counting are crucial tools for making informed decisions in various aspects of daily life and professional practice.

This Story also Contains

Fundamental principle of counting
Multiplication Rule: Definition
Proof of multiplication rule of the fundamental principle of counting
Addition Rule: Definition
Solved Examples Based on the Fundamental Principles of Counting

Fundamental Principle of Counting

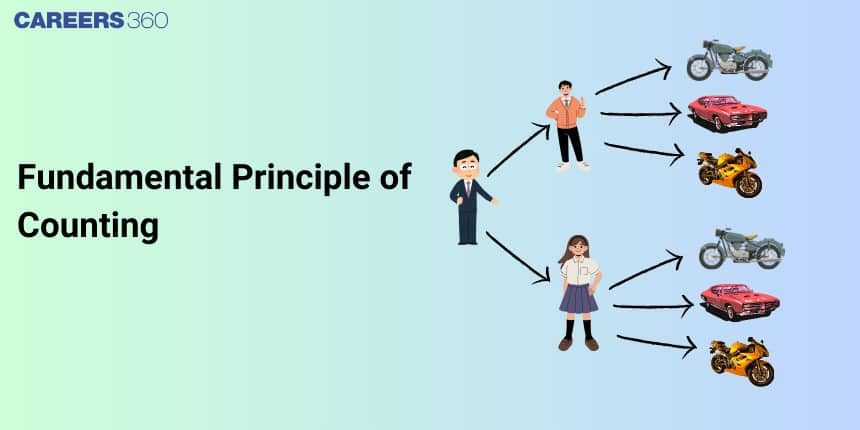
In this article, we will learn about the fundamental principles of counting. This topic falls under the broader category of Permutations and combinations, which is a crucial chapter in Class 11 Mathematics. This is very important not only for board exams but also for competitive exams, which even include the Joint Entrance Examination Main and other entrance exams: SRM Joint Engineering Entrance, BITSAT, WBJEE, and BCECE. A total of thirty questions have been asked on this topic in JEE Main from 2013 to 2023 including two in 2022 and three in 2023.

Fundamental principle of counting

The fundamental principle of counting is a rule used to find the total number of outcomes possible in a given situation. The fundamental principle of counting can be classified into two types

Multiplication Rule (AND rule)
Addition Rule (OR rule)

Multiplication Rule: Definition

The multiplication Rule states that for “n” mutually independent events, P₁, P₂, P₃, …P_n. The number of in which these events can occur is n(P₁), n(P₂), n(P₃),… n(P_n) respectively. Now we define an event E such that it is happening all the events simultaneously then the number of ways this can happen is, n( E) = n(P₁) x n(P₂).........................n(P_n)

NEET Highest Scoring Chapters & Topics

This ebook serves as a valuable study guide for NEET exams, specifically designed to assist students in light of recent changes and the removal of certain topics from the NEET exam.

Download E-book

Formula for Multiplication Rule

According to the multiplication rule, if a certain work W can be completed by doing 2 tasks, first doing task A AND then doing task B. A can be done in m ways and following that B can be done in n ways, then the number of ways of doing the work W is (m x n) ways.

For example, let's say a person wants to travel from Noida to Gurgaon, and he has to travel via New Delhi. It is given that the person can travel from Noida to New Delhi in 3 different ways and from New Delhi to Gurgaon in 5 different ways.

So, in this case, to complete his work (reach Gurgaon) he has to do two tasks one after the other, first traveling from Noida to New Delhi (task A) and then from New Delhi to Gurgaon (task B), as he has 3 different ways of reaching New Delhi (doing task A), and he has 5 different ways to reach Gurgaon from New Delhi (doing task B), so in that way, he has a total of 3×5 = 15 different ways to reach Gurgaon from Noida.

Proof of multiplication rule of the fundamental principle of counting

The first operation can be performed in any one of the m ways and for each of these ways of performing the first operation, there are n ways of performing the second operation.

Thus, if the first operation could be performed in one such way, there would have been 1 x n = n ways of performing both operations. But it is given that the first operation can be performed in m ways and for each way of performing the first operation, the second can be performed in n ways.

Therefore, the total number of ways of performing both operations is n + n + n +… to m terms = n x m.

Note: If three operations can be separately performed in m, n, and p ways, respectively, then the three operations together can be performed in mx n x p ways.

Addition Rule: Definition

The addition Rule states that for two possible events A and B where A and B both are mutually exclusive events, i.e. they have no outcome in common, and if event E is defined as occurring in either event A or event B then the possible number of ways in which event E can occur is n(E) = n(A) + n (B)

Formula for Addition Rule

According to the addition rule, if work W can be completed by doing task A OR task B, and A can be done in m ways and B can be done in n ways (and both cannot occur simultaneously: in this case, we call tasks A and B as mutually exclusive), then work W can be done in (m + n) ways.

Suppose, there are 5 doors in a room: 2 on one side and 3 on the other. A man has to go out of the room. The man can go out from any one of the 5 doors. Thus, the number of ways in which the man can go out is 5. Here, the work of going out through the doors on one side will be done in 2 ways and the work of going out through the doors on the other side will be done in 3 ways. The work of going out will be done when the man goes out from either side I or side II. Thus, the work of going out can be done in 2 + 3 = 5 ways.

For example, let’s say that a person can travel from New Delhi to Noida in 3 different types of buses, and 2 different types of trains, so, he can complete the work of going from New Delhi to Noida in 3 + 2 = 5 ways (As work can be completed by going by bus (A) OR by going by train (B))

Solved Examples Based on the Fundamental Principles of Counting

Example 1: How many ways are there to write a 4-digit positive integer using the digits 2, 3, 4, 6, and 8 if no digit is used more than once?

Solution: Since we can choose from the five available digits, we have five options for the first digit.

Similarly, because we have used up one of the digits, there are four options for the second digit and three options for the third digit.

Again 2 options are available for the fourth digit.

So the total number of ways to write a 4-digit positive integer using the digits 2, 3, 4, 6, and 8 is

$5 \times 4 \times 3 \times 2=120$

Hence, the required answer is 120.

Example 2: In a race, there are 10 participants. In how many ways can the gold, silver, and bronze medals be awarded?

Solution: The gold medal can be awarded to any one of the 10 participants, so there are 10 choices for the gold medalist.

After the gold medalist is determined, there are 9 remaining participants who could receive the silver medal. Therefore, there are 9 choices for the silver medalist.

Finally, after the gold and silver medals are assigned, there are 8 remaining participants who could receive the bronze medal. Thus, there are 8 choices for the bronze medalist.

To find the total number of ways, we multiply the number of choices for each medal: 10 choices × 9 choices × 8 choices = 720 ways.

Hence, the required answer is 720.

Example 3: The number of three-digit even numbers, formed by the digits 0,1,3,4,6,7 if the repetition of digits is not allowed, is _________

Solution:

Case 1: when 0 is at the unit's place $5 \times 4 \times 1=20$
Case 2: when 0 is not at the unit's place $4 \times 4 \times 2=32$

Total $=20+32=52$

Hence, the required answer is 52

Example 4: A class teacher wishes to assign one question from each of the two exercises in a book. If the two exercises have 20 and 15 questions, respectively, how many ways can the two questions be chosen?

Solution: Given that,

A class teacher wants to assign 2 questions from two exercises.

The first exercise has 20 questions. So, there are 20 possible ways to choose a question.

The second exercise has 15 questions. So, there are 15 possible ways to choose a question.

Thus, using the fundamental counting principle the 2 questions can be answered in $20 \times 15=300$ ways.

Hence, the required answer is 300.

Example 5: A women's fashion store sells 6 different kurtas, 5 different skirts, 2 different tops, and 4 different pairs of pants. How many different suits consisting of kurtas, skirts, tops, and pants are possible?

Solution:

Given that,

A women's fashion store sells 6 different kurtas, 5 different skirts, 2 different tops, and 4 different pairs of pants.

Thus,

$\begin{aligned} & \mathrm{n}=6 \times 5 \times 2 \times 4 \\ & \mathrm{n}=240\end{aligned}$

Hence, the required answer is 240.

Frequently Asked Questions (FAQs)

1. How does the Fundamental Principle of Counting help in understanding the concept of exponential growth?

The Fundamental Principle of Counting directly relates to exponential growth. When we have n independent choices, each with m options, the total number of possibilities is m^n. This exponential relationship is at the core of understanding exponential growth in various fields, from population biology to compound interest calculations.

2. What role does the Fundamental Principle of Counting play in combinatorial proofs?

The Fundamental Principle of Counting is often used in combinatorial proofs to establish bijections between sets. By showing that two different counting methods yield the same result, we can prove combinatorial identities and relationships between different counting formulas.

3. How does the Fundamental Principle of Counting help in understanding the concept of coding theory?

In coding theory, the Fundamental Principle of Counting is used to determine the number of possible messages of a given length. For a code with alphabet size q and message length n, there are q^n possible messages, which is a direct application of the principle.

4. How can the Fundamental Principle of Counting be used to solve problems involving divisibility?

In divisibility problems, the Fundamental Principle of Counting can be used to count the number of integers with certain properties. For example, to count numbers divisible by both 2 and 3 within a range, we can use the principle to combine the conditions of divisibility by 2 and by 3.

5. Can the Fundamental Principle of Counting be applied to problems involving non-uniform probability distributions?

While the Fundamental Principle of Counting assumes equal probability for each outcome, it can be adapted for non-uniform distributions by weighting the counts. This leads to more advanced concepts like expected value and weighted averages in probability theory.

6. How can the Fundamental Principle of Counting be used to solve problems involving combinations with repetition?

For combinations with repetition, the Fundamental Principle of Counting is used indirectly. The problem is often transformed into an equivalent problem of distributing indistinguishable objects into distinct boxes, where the principle can be applied more directly.

7. How can the Fundamental Principle of Counting be used to solve problems involving derangements?

While the Fundamental Principle of Counting doesn't directly solve derangement problems (where no element is in its original position), it's used in deriving the formula for derangements. The principle helps in breaking down the problem into cases and counting the complement of derangements.

8. What's the difference between using the Fundamental Principle of Counting and using formulas for permutations and combinations?

The Fundamental Principle of Counting is a more general concept that can be applied to a wide range of problems. Formulas for permutations and combinations are derived from this principle but are more specific and can be applied directly to certain types of counting problems without having to break down the problem into individual choices.

9. How can the Fundamental Principle of Counting help in solving problems involving restrictions?

When solving problems with restrictions, the Fundamental Principle of Counting can be applied by breaking down the problem into steps that satisfy the restrictions. For each step, we determine the number of valid choices, then multiply these numbers together to get the total count of valid outcomes.

10. Can the Fundamental Principle of Counting be applied to problems involving repetition?

Yes, the Fundamental Principle of Counting can be applied to problems involving repetition. In such cases, instead of decreasing the number of available choices for each subsequent selection (as in permutations without repetition), the number of choices remains constant for each selection.

11. What's the difference between dependent and independent events in counting problems?

Independent events are those where the occurrence of one event doesn't affect the probability of the other events. The Fundamental Principle of Counting applies directly to independent events. Dependent events, on the other hand, are influenced by the outcomes of other events, and require a modified approach to counting.

12. How does the Fundamental Principle of Counting relate to the multiplication rule in probability?

The Fundamental Principle of Counting is essentially the same as the multiplication rule in probability for independent events. Both state that the probability (or number of ways) of multiple independent events occurring together is the product of their individual probabilities (or number of ways).

13. Can the Fundamental Principle of Counting be applied to problems involving conditional probability?

While the Fundamental Principle of Counting primarily deals with independent events, it can be adapted for conditional probability problems. In such cases, we apply the principle sequentially, adjusting the number of possibilities at each step based on the given conditions.

14. What role does the Fundamental Principle of Counting play in understanding combinatorial game theory?

In combinatorial game theory, the Fundamental Principle of Counting helps in analyzing the number of possible game states or moves. It's used to calculate the game tree complexity and understand the growth of possible game scenarios as the game progresses.

15. How can the Fundamental Principle of Counting be used to solve problems involving partitions of sets?

While the Fundamental Principle of Counting doesn't directly solve partition problems, it's used in deriving formulas for the number of ways to partition a set. It helps in breaking down the problem into steps and counting the number of choices at each step.

16. How does the Fundamental Principle of Counting help in understanding the concept of dimensional analysis?

In dimensional analysis, the Fundamental Principle of Counting helps in understanding how different units combine. Each dimension (like length, time, mass) can be thought of as an independent choice, and the principle helps in determining how many different combinations of base units can form a derived unit.

17. What's the connection between the Fundamental Principle of Counting and the concept of entropy in information theory?

The Fundamental Principle of Counting relates to entropy in information theory as both deal with the number of possible states or outcomes. Entropy measures the average amount of information in a set of possible outcomes, which is directly related to the number of these outcomes as calculated by the counting principle.

18. What role does the Fundamental Principle of Counting play in understanding the concept of sample size in statistics?

The Fundamental Principle of Counting helps in understanding the concept of sample size by illustrating how quickly the number of possible samples grows as the sample size increases. This understanding is crucial for concepts like statistical power and confidence intervals.

19. Can the Fundamental Principle of Counting be applied to problems involving conditional arrangements?

Yes, the Fundamental Principle of Counting can be applied to conditional arrangements by breaking the problem into steps. For each step, we count the number of valid choices given the conditions, then multiply these numbers together to get the total count of valid arrangements.

20. Can the Fundamental Principle of Counting be applied to problems involving overlapping sets?

While the Fundamental Principle of Counting doesn't directly handle overlapping sets, it's used in conjunction with the Inclusion-Exclusion Principle to solve such problems. The principle helps in counting the size of each set and their intersections.

21. How does the Fundamental Principle of Counting relate to the concept of degrees in graph theory?

In graph theory, the Fundamental Principle of Counting can be used to calculate the number of possible graphs with a given number of vertices and degrees. It helps in understanding how many ways edges can be distributed among vertices while maintaining specific degree requirements.

22. What's the connection between the Fundamental Principle of Counting and the concept of spanning trees in graph theory?

The Fundamental Principle of Counting is used in deriving formulas for counting spanning trees, such as Cayley's formula. It helps in breaking down the process of constructing a spanning tree into a series of independent choices.

23. What is the Fundamental Principle of Counting?

The Fundamental Principle of Counting states that if one event can occur in 'm' ways, and another independent event can occur in 'n' ways, then the two events can occur together in 'm × n' ways. This principle forms the basis for solving more complex counting problems and is essential in understanding permutations and combinations.

24. How does the Fundamental Principle of Counting relate to real-life scenarios?

The Fundamental Principle of Counting applies to many real-life situations where we need to count the total number of possible outcomes. For example, if you have 5 shirts and 3 pairs of pants, the principle tells us you have 5 × 3 = 15 different outfit combinations. It helps in decision-making, probability calculations, and organizing events.

25. Can the Fundamental Principle of Counting be applied to more than two events?

Yes, the Fundamental Principle of Counting can be extended to any number of independent events. If you have events A, B, C, and D with m, n, p, and q ways of occurring respectively, the total number of ways all events can occur together is m × n × p × q.

26. How does the Fundamental Principle of Counting relate to tree diagrams?

Tree diagrams visually represent the Fundamental Principle of Counting. Each branch in the diagram represents a possible outcome for an event, and the number of end points on the tree represents the total number of possible outcomes, which is the product of the number of branches at each level.

27. What's the connection between the Fundamental Principle of Counting and factorial notation?

The Fundamental Principle of Counting is closely related to factorial notation. For instance, when arranging n distinct objects, we have n choices for the first position, (n-1) for the second, and so on. Multiplying these numbers according to the principle gives us n!, which is the factorial of n.

28. How does the Fundamental Principle of Counting relate to the concept of state spaces in computer science?

In computer science, state spaces represent all possible states a system can be in. The Fundamental Principle of Counting helps in calculating the size of these state spaces by multiplying the number of possibilities for each independent variable or component of the system.

29. How can the Fundamental Principle of Counting be used to solve problems involving permutations with constraints?

For permutations with constraints, the Fundamental Principle of Counting is applied sequentially. We break the problem into steps, count the number of valid choices for each step given the constraints, then multiply these numbers together to get the total count of valid permutations.

30. How can the Fundamental Principle of Counting be used to solve problems involving permutations with repetition?

For permutations with repetition, the Fundamental Principle of Counting is applied directly. If we have n positions to fill and k types of objects (with repetition allowed), we have k choices for each position, resulting in k^n total permutations.

31. How does the Fundamental Principle of Counting help in understanding permutations?

The Fundamental Principle of Counting is the foundation for understanding permutations. In a permutation, we arrange objects in a specific order. The principle helps us calculate the number of ways to do this by multiplying the number of choices available for each position in the arrangement.

32. How does the Fundamental Principle of Counting help in understanding the concept of permutation groups in abstract algebra?

The Fundamental Principle of Counting is used to determine the size of permutation groups. For instance, the size of the symmetric group on n elements (all permutations of n objects) is n!, which is derived directly from applying the principle to arrange n distinct objects.

33. Can the Fundamental Principle of Counting be applied to problems involving circular arrangements?

Yes, but with a modification. In circular arrangements, rotations of the same arrangement are considered identical. To account for this, we typically use the Fundamental Principle of Counting to calculate the total number of linear arrangements, then divide by the number of positions (which represents the number of rotations that produce the same circular arrangement).

34. How does the Fundamental Principle of Counting relate to the concept of independence in probability?

The Fundamental Principle of Counting assumes that the events or choices are independent, meaning the outcome of one doesn't affect the others. This directly parallels the concept of independence in probability, where the probability of one event occurring doesn't affect the probability of another independent event.

35. How does the Fundamental Principle of Counting relate to the concept of complexity in algorithm analysis?

In algorithm analysis, the Fundamental Principle of Counting helps in determining the number of operations an algorithm performs. For nested loops or recursive calls, the principle is used to calculate the total number of iterations or recursive steps, which directly relates to the algorithm's time complexity.

36. What's the relationship between the Fundamental Principle of Counting and the addition principle?

While the Fundamental Principle of Counting (multiplication principle) deals with combining independent choices, the addition principle is used when we have mutually exclusive events and we want to count the total number of ways these events can occur. Both principles are fundamental to combinatorics and can be used together to solve complex counting problems.

37. How does the Fundamental Principle of Counting relate to the concept of sample space in probability?

The Fundamental Principle of Counting is often used to calculate the size of a sample space in probability problems. The sample space is the set of all possible outcomes, and its size is often determined by multiplying the number of possibilities for each independent component of the experiment.

38. Can the Fundamental Principle of Counting be applied to problems involving "at least" or "at most" conditions?

Yes, but indirectly. For "at least" or "at most" problems, it's often easier to count the complement of the desired set using the Fundamental Principle of Counting, then subtract from the total number of possibilities to get the desired count.

39. What role does the Fundamental Principle of Counting play in cryptography?

In cryptography, the Fundamental Principle of Counting is crucial for determining the number of possible keys or codes. For instance, if a password consists of 8 characters, each of which can be one of 94 possible characters, the total number of possible passwords is 94^8, calculated using the principle.

40. What's the connection between the Fundamental Principle of Counting and the multiplication principle in computer science?

In computer science, the multiplication principle (which is another name for the Fundamental Principle of Counting) is used in algorithm analysis to determine the number of operations or possibilities in nested loops or recursive functions. It helps in calculating time complexity and understanding the growth of algorithms.

41. What role does the Fundamental Principle of Counting play in understanding the concept of orthogonality in mathematics?

While not directly related, the Fundamental Principle of Counting and orthogonality both deal with independence. In counting, we multiply independent choices; in orthogonality, we deal with independent directions or functions. This parallel helps in understanding both concepts more deeply.

42. How does the Fundamental Principle of Counting relate to the concept of cartesian product in set theory?

The Fundamental Principle of Counting is essentially a way to count the number of elements in a Cartesian product of sets. If set A has m elements and set B has n elements, their Cartesian product A × B will have m × n elements, which is exactly what the principle states.

43. What's the connection between the Fundamental Principle of Counting and the concept of degrees of freedom in statistics?

The Fundamental Principle of Counting relates to degrees of freedom in that both concepts deal with the number of independent choices or variables in a system. In statistics, degrees of freedom often represent the number of independent pieces of information available, which is analogous to the number of independent choices in a counting problem.

44. What's the connection between the Fundamental Principle of Counting and the concept of basis in linear algebra?

The Fundamental Principle of Counting relates to the concept of basis in linear algebra in that both deal with independent choices. The number of elements in a basis (the dimension of the space) is analogous to the number of independent choices in a counting problem.

45. How does the Fundamental Principle of Counting help in understanding the concept of linear independence in linear algebra?

The Fundamental Principle of Counting relates to linear independence in that both concepts deal with the idea of independent choices or elements. In linear algebra, linear independence ensures that each vector contributes a new dimension, which is analogous to each choice being independent in a counting problem.

46. How does the Fundamental Principle of Counting relate to the concept of basis vectors in vector spaces?

The Fundamental Principle of Counting is analogous to how basis vectors span a vector space. Each basis vector represents an independent direction, much like each independent choice in a counting problem. The number of basis vectors (dimension of the space) is similar to the number of independent choices in the counting principle.

47. What role does the Fundamental Principle of Counting play in understanding the concept of information content in data compression?

The Fundamental Principle of Counting helps in understanding the theoretical limits of data compression. The principle is used to calculate the number of possible messages of a given length, which relates directly to the minimum number of bits needed to represent these messages.

48. What's the connection between the Fundamental Principle of Counting and the concept of degrees of freedom in physics?

The Fundamental Principle of Counting relates to degrees of freedom in physics in that both deal with independent variables or choices. In physics, degrees of freedom represent the number of independent parameters needed to describe a system, which is analogous to the number of independent choices in a counting problem.