Geometric Mean In GP - Definition and Properties

Mean is the average of the given data set. The different types of mean are Arithmetic mean (AM), Geometric mean(GM), and Harmonic Mean (HM). The Geometric mean is the average value or mean which signifies the central tendency of the set of numbers by finding the product of their values. In real life, we use Geometric mean in electrical circuits, population growth, growth of bacteria, and amortization of loans.

In this article, we will cover the concept of Geometric mean. This category falls under the broader category of Matrices, which is a crucial Chapter in class 11 Mathematics. It is essential not only for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of JEE Main Exam (from 2013 to 2023), a total of fourteen questions have been asked on this concept including one in 2020 and one in 2022.

Geometric Sequence

A geometric sequence is a sequence where the first term is non-zero and the ratio between consecutive terms is always constant. The ‘constant factor’ is called the common ratio and is denoted by ‘r’. r is also a non-zero number.

The first term of a G.P. is usually denoted by 'a'. If $a_1, a_2, a_3 \ldots . a_{n-1}, a_n$ is in geometric progression then, $r=\frac{a_2}{a_1}=\frac{a_3}{a_2}=\ldots=\frac{a_n}{a_{n-1}}$

Eg ,
- $2,6,18,54, \ldots .(a=2, r=3)$
- $4,2,1,1 / 2,1 / 4, \ldots .(a=4, r=1 / 2)$
- $-5,5,-5,5, \ldots \ldots(a=-5, r=-1)$

General Term of a GP

$
\begin{aligned}
& a_1=a=a r^{1-1}\left(1^{\text {st }} \text { term }\right) \\
& a_2=a r=a r^{2-1}\left(2^{\text {nd }} \text { term }\right) \\
& a_3=a r^2=a r^{3-1}\left(3^{\text {rd }} \text { term }\right) \\
& \cdots \\
& \cdots \\
& a_n=a r^{n-1}\left(\mathrm{n}^{\text {th }} \text { term }\right)
\end{aligned}
$
So, the general term or $\mathrm{n}^{\text {th }}$ term of a geometric progression is $a_n=a r^{n-1}$

Geometric Mean

If three terms are in G.P., then the middle term is called the Geometric Mean (G.M.) of the other two numbers. So if $a, b$, and $c$ are in G.P., then b is GM of a and c ,

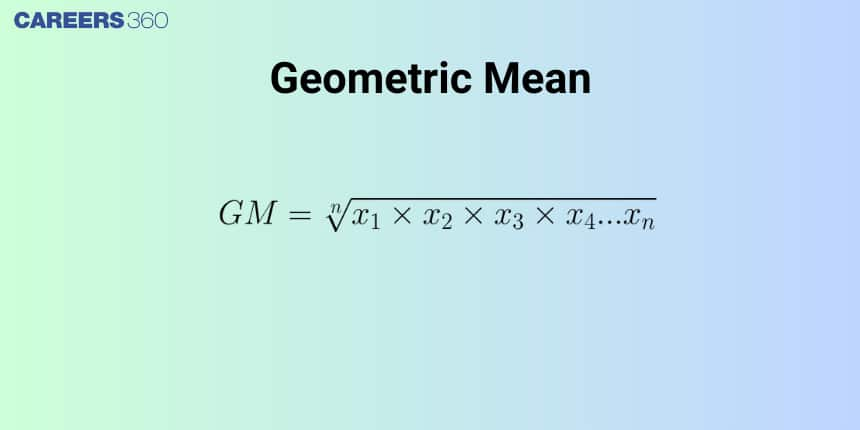
If $a_1, a_2, a_3, \ldots . ., a_n$ are n positive numbers, then the Geometric Mean of these numbers is given by $G=\sqrt[n]{a_1 \cdot a_2 \cdot a_3 \cdot \ldots \cdot \cdot a_n}$.

If a and b are two numbers and G is the GM of a and b . Then, $\mathrm{a}, \mathrm{G}$, and b are in geometric progression.

Hence, $G=\sqrt{a \cdot b}$
Insertion of n-Geometric Mean Between a and b
Let $r$ be the common ratio of this GP.
now, $\mathrm{b}=(\mathrm{n}+2)^{\text {th }}$ term $=\mathrm{ar}^{\mathrm{n}+2-1}$
$\therefore r=\left(\frac{b}{a}\right)^{\frac{1}{n+1}}$
[where, $\mathrm{r}=$ common ratio]
$\therefore \mathrm{G}_1=$ ar, $\mathrm{G}_2=\operatorname{ar}^2, \mathrm{G}_3=\operatorname{ar}^3, \ldots ., \mathrm{Ga}_{\mathrm{n}}=\operatorname{ar}^{\mathrm{n}}$
$\Rightarrow \mathrm{G}_1=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{1}{\mathrm{n}+1}}, \mathrm{G}_2=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{2}{\mathrm{n}+1}}, \mathrm{G}_3=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{3}{\mathrm{n}+1}} \ldots \ldots$
$\mathrm{G}_{\mathrm{n}}=\mathrm{a}\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{\mathrm{n}}{\mathrm{n}+1}}$

Important Property of GM

The product of $n$ geometric mean between $a$ and $b$ is equal to the $n^{t h}$ power of a single geometric mean between $a$ and $b$.
If a and b are two numbers and $\mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3 \ldots, \mathrm{G}_{\mathrm{n}}$ are n -geometric mean between a and b , then $a, \mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3 \ldots, \mathrm{G}_{\mathrm{n}}, b$ will be in geometric progression.
So, the Product of n-G.M's between a and b is

$
\begin{aligned}
& \mathrm{G}_1 \cdot \mathrm{G}_2 \cdot \mathrm{G}_3 \cdot \ldots \cdot \mathrm{G}_{\mathrm{n}}=(a r)\left(a r^2\right)\left(a r^3\right) \ldots\left(a r^n\right) \\
& \Rightarrow\left(a^{1+1+1+\ldots \mathrm{n}-\text { times }}\right)\left(r^{1+2+3+\ldots+n}\right) \\
& \Rightarrow a^n\left(r^{\left(\frac{n(n+1)}{2}\right)}\right) \quad\left(1+2+\ldots . \cdot+n=\frac{n(n+1)}{2} U \text { sing sum of } A P\right) \\
& \text { replace } r \text { with }\left(\frac{\mathrm{b}}{\mathrm{a}}\right)^{\left(\frac{1}{\mathrm{n}+1}\right)} \\
& \Rightarrow a^n \cdot\left[\left(\frac{b}{a}\right)^{\frac{1}{n+1}}\right]^{\frac{n(n+1)}{2}}=a^n\left(\frac{b}{a}\right)^{\frac{n}{2}} \\
& \Rightarrow(a)^{\frac{n}{2}}(b)^{\frac{n}{2}}=(\sqrt{a \cdot b})^n \\
& =[\mathrm{G} \cdot \mathrm{M} . \text { of } a \text { and } b]^{\mathrm{n}}
\end{aligned}
$

Recommended Video Based on Geometric Mean

Solved Examples Based on Geometric Mean

Example 1: Consider two G.Ps. $2,2^2, 2^3, \ldots$ and $4,4^2, 4^3, \ldots$ of 60 and ' $n$ ' terms respectively. If the geometric mean of all the $60+n$ terms is $\qquad$ is equal to:

[JEE
MAINS 2022]
Solution: Given: $\left(\left(2^1 \cdot 2^2 \cdots \cdots 2^{60}\right)\left(4^1 \cdot 4^2 \cdots 4^{\mathrm{n}}\right)\right)^{\frac{1}{60+n}}=2^{\frac{225}{8}}$

$
\begin{aligned}
& \Rightarrow\left(2^{30 \times 61} \cdot 4^{\frac{\mathrm{n}(\mathrm{n}+1)}{2}}\right)^{\frac{1}{60+n}}=2^{\frac{225}{8}} \\
& \Rightarrow 2^{1830+\mathrm{n}^2+\mathrm{n}}=2^{\frac{(225)(60+\mathrm{n})}{8}} \\
& \Rightarrow 8 \mathrm{n}^2-217 \mathrm{n}+1140=0 \\
& \Rightarrow \mathrm{n}=20, \frac{57}{8}
\end{aligned}
$

Now,

$
\begin{aligned}
\sum_{k=1}^n n k-k^2 & =\frac{n^2(n+1)}{2}-\frac{n(n+1)(2 n+1)}{6} \\
& =1330
\end{aligned}
$

Hence, the answer is 1330

Example 2: If $m$ arithmetic means $(A . M s)$ and three geometric means $(G . M s)$ are inserted between 3 and 243 such that $4^{\text {th }}$ A.M. is equal to $2^{\text {nd }}$ G.M., then $m$ is equal to $\qquad$
[JEE MAINS 2020]

Solution: Let $\mathrm{A}_1, \mathrm{~A}_2, \mathrm{~A}_3 \ldots, \mathrm{A}_{\mathrm{n}}$ be n arithmetic mean between two numbers a and b . Then, $a, \mathrm{~A}_1, \mathrm{~A}_2, \mathrm{~A}_3 \ldots, \mathrm{A}_{\mathrm{n}}, b$ is an A.P. Let d be the common difference of this A.P. Clearly, this A.P. contains $n+2$ terms, and

Let $\mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3 \ldots, \mathrm{G}_{\mathrm{n}}$ be n geometric mean between two numbers a and b . Then, $a, \mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3 \ldots, \mathrm{G}_{\mathrm{n}}, b$ is a G.P. Clearly, this G.P. contains $\mathrm{n}+2$ terms.

Given,
$3, \mathrm{~A}_1, \mathrm{~A}_2 \ldots \ldots . \mathrm{A}_{\mathrm{m}}, 243 \Rightarrow \mathrm{d}=\frac{243-3}{\mathrm{~m}+1}=\frac{240}{\mathrm{~m}+1}$ Now $3, \mathrm{G}_1, \mathrm{G}_2, \mathrm{G}_3, 243$

$
\begin{aligned}
& \Rightarrow \mathrm{r}=\left(\frac{243}{3}\right)^{\frac{1}{3+1}}=3 \\
\therefore \quad & \mathrm{A}_4=\mathrm{G}_2 \\
\Rightarrow \quad & \mathrm{a}+4 \mathrm{~d}=\mathrm{ar}^2 \\
\Rightarrow & 3+4\left(\frac{240}{\mathrm{~m}+1}\right)=3(3)^2 \\
& \Rightarrow \mathrm{m}=39
\end{aligned}
$

Hence, the answer is 39

Example 3: If the arithmetic mean of two numbers a and $b, a>b>0$, is

$
\frac{a+b}{a-b}
$

is equal to:
five times their geometric mean, then $a$

$
A=\frac{a+b}{2}
$

and

The geometric mean of two numbers (GM) $-G M=\sqrt{a b}$
In this Question,

$
\begin{aligned}
& \frac{a+b}{2}=5 \sqrt{a b} \\
& a+b=10 \sqrt{a b}
\end{aligned}
$

We know,

$
\begin{aligned}
& (a-b)^2=(a+b)^2-4 a b \\
& =(10 \sqrt{a b})^2-4 a b=100 a b-4 a b=96 a b \\
& a-b=4 \sqrt{6} \sqrt{a b}
\end{aligned}
$
Therefore:

$
\frac{a+b}{a-b}=\frac{10 \sqrt{a b}}{4 \sqrt{6} \sqrt{a b}}=5 \frac{\sqrt{6}}{12}
$

Hence, the answer is $\frac{5 \sqrt{6}}{12}$

Example 4: Let G be the geometric mean of two positive numbers a and b , and M be the arithmetic mean of $\frac{1}{a}$ and $\frac{1}{b}$ if $\frac{1}{M}: G$ is $4: 5$ then a:b can be:

Solution: We know that the arithmetic mean of two numbers (AM) is given by $A=\frac{a+b}{2}$
and

The geometric mean of two numbers (GM) is given by $G M=\sqrt{a b}$
Now, given $\mathrm{G}^2=\mathrm{ab}$

$
\begin{aligned}
& 2 M=\frac{1}{a}+\frac{1}{b} \\
& \text { and } \frac{\frac{1}{M}}{G}=\frac{4}{5} \\
& \therefore G M=\frac{5}{4}
\end{aligned}
$

Squaring both sides we get,

$
\therefore G^2 M^2=\frac{25}{16}
$

Putting values of G and M ,

$
\begin{aligned}
& a b \times\left(\frac{a+b}{2 a b}\right)^2=\frac{25}{16} \\
& \frac{a b \times(a+b)^2}{4 a^2 b^2}=\frac{25}{16}
\end{aligned}
$

solving equation we get,

$
\therefore 4 a^2+4 b^2-17 a b=0
$

dividing the equation by $b$

$
\therefore 4\left(\frac{a}{b}\right)^2-17\left(\frac{a}{b}\right)+4=0
$

So, we get

$
\therefore \frac{a}{b}=1: 4
$

Hence, the answer is 1:4

Example 5: If $\alpha, \beta$ and $\gamma$ are three consecutive terms of a nonconstant G.P. such that the equations $\alpha x^2+2 \beta x+\gamma=0$ and $x^2+x-1=0$ have a common root, then $\alpha(\beta+\gamma)$ is equal to:

Solution: The geometric mean of two numbers (GM) is given by $G M=\sqrt{a b}$

Condition for Real and equal roots of Quadratic Equation -

$
D=b^2-4 a c=0
$

Now,
$\alpha, \beta$ and $\gamma$ are in G.P. $\Rightarrow \beta^2=\alpha \gamma$
For equation, $\alpha x^2+2 \beta x+\gamma=0$

$
\Delta=4 \beta^2-4 \alpha \gamma=0
$

Hence, roots are equal \& equal to $-\frac{\beta}{\alpha}$ (Using the sum of roots)

$
-\frac{\beta}{\alpha}=-\frac{\gamma}{\beta}(\text { Using GP relation) }
$

Since the given equation has common roots, hence $-\frac{\gamma}{\beta}$ must be the root of $x^2+x-1=0$

$
\begin{aligned}
& =>\frac{\gamma^2}{\beta^2}-\frac{\gamma}{\beta}-1=0 \\
& =>\gamma^2-\gamma \beta-\beta^2=0 \\
& =>\gamma^2=\beta(\gamma+\beta) \\
& =>\gamma \cdot \frac{\beta^2}{\alpha}=\beta(\gamma+\beta) \\
& =>\gamma \cdot \beta=\alpha(\gamma+\beta)
\end{aligned}
$

Hence, the answer is $\beta \gamma$