Limits

In calculus, limits are crucial for understanding the behavior of functions as they approach specific points. Mastering limits is key to grasping fundamental calculus concepts. They help analyze changes, optimize processes, and predict trends in fields like engineering, physics, and economics. Limits allow us to study functions near critical points, even if the function is not defined at those points. This foundational concept is essential for solving complex real-world problems and mathematical equations, such as finding derivatives and integrals.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. What is a Limit?
2. Solved Examples Based on Limits
3. Summary

In this article, we will cover the concept of Limits. This topic falls under the broader category of Calculus, which is a crucial chapter in Class 11 Mathematics. It is essential for board exams and competitive exams like the Joint Entrance Examination (JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, and BCECE. From 2013 to 2023, a total of three questions have been asked on JEE Main from this topic in 2023.

What is a Limit?

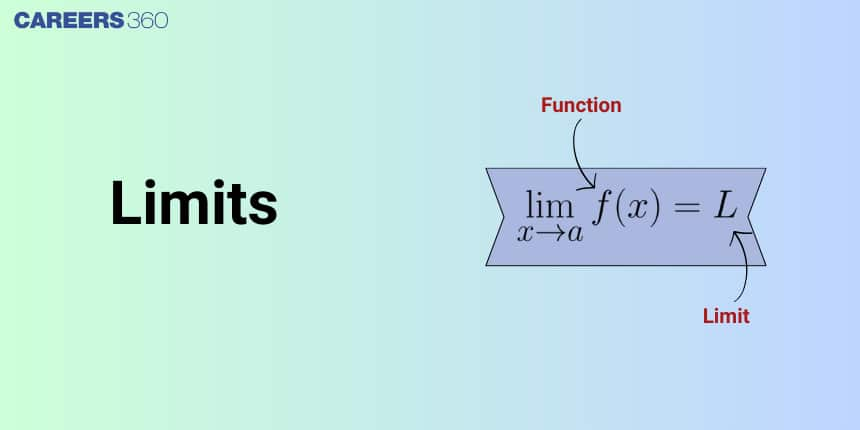
A limit describes the value that a function $f(x)$ approaches as the variable $x$ approaches a particular point $a$.

In formulas, a limit of a function is usually written as $\lim\limits_{x \to c} f(x) = L$, and is read as the limit of $f$ of $x$ as $x$ approaches $c$ equals $L$.

Let's consider the function $\mathrm{f}({x})={x}^2$

Observe that as $x$ takes values very close to $0$, the value of $f(x)$ is also close to $0$. (See graph below)

We can also interpret it in another way. If we input the values of $x$ which tend to approach $0$ (meaning close to $0$, either just smaller than $0$ or just larger than $0$ ), the value of $f(x)$ will tend to approach $0$(meaning close to $0$, either just smaller than $0$ or just larger than $0$).

Then we can say that, $\lim\limits_{x \to 0} f(x) = 0$

Similarly, when $x$ approaches $2$, the value of $f(x)$ approaches $4$, i.e. $\lim\limits_{x \to 2} f(x) = 4$ or $\lim\limits_{x \to 2} x^2 = 4$.

In general, as $x \to a$ , $f(x) \to l$, then $l$ is called the limit of the function $\mathrm{f}(\mathrm{x})$, which is symbolically written as $\lim\limits_{x \to a} f(x) = l$.

Now consider the function

$
f(x)=\frac{x^2-6 x-7}{x-7}
$
We can factor the function as shown
$f(x)=\frac{(x-7)(x+1)}{x-7} \quad$ [Cancel like factors in numerator and denominator.]

$
f(x)=x+1, x \neq 7
$

Notice that $x$ cannot be $7$, or we would be dividing by $ 0$ , so $7$ is not in the domain of the original function. To avoid changing the function when we simplify, we set the same condition, $x \neq 7$, for the simplified function. We can represent the function graphically

What happens at $x=7$ completely differs from what happens at points close to $x=7$ on either side. Just observe that as the input $x$ approaches $7$ from either the left or the right, the output approaches $8$. The output can get as close to $8$ as we like if the input is sufficiently near $7$ . So we say that the limit of this function at $x=7$ equals $8$.

So even if the function does not exist at x = a, still the limit can exist at that point as the limit is concerned only about the points close to $x=a$ and NOT at $x=a$ itself.

Some Properties of Limits:

• $\lim\limits_{\mathrm{x} \to \mathrm{a}} \mathrm{c} = \mathrm{c}$, where $c$ is a constant quantity.
• The value of $\lim\limits_{\mathrm{x} \to \mathrm{a}} {x} = \mathrm{a}$.
• Value of $\lim\limits_{x \to a} (b x + c) = b a + c$.
• $\lim\limits_{x \to a} x^n = a^n$, if $n$ is a positive integer.

Recommended Video Based on Limits

Solved Examples Based on Limits

Example 1: Which of the following is incorrect?

(1) As $x$ approaches $2$, tends to reach $4$.
2) As $x$ approaches tends to reach $0$.
3) As $x$ approach tends to reach $\infty$.

4) As $x$ approach $\frac{\pi}{2}$, then $\tan x$ has a tendency to reach $\infty$.

Solution:
Limits describe the behaviour of a function $f(x)$ as its variable $x$ approaches a particular number.

1) $\lim\limits_{x \to 2} x^2 = 4$ — Statement 1 is correct.

2) $\lim\limits_{x \to \pi} \sin x = 0$ — Statement 2 is correct.

3) $\lim\limits_{x \to \pi / 2} \sin x = 1$ — Statement 3 is incorrect.

4) $\lim\limits_{x \to \pi / 2} \tan x = \infty$ — Statement 4 is correct.
Hence, the answer is the option 3. $\qquad$

Example 2: $\lim _{x \rightarrow 0}\left(\left(\frac{\left(1-\cos ^2(3 x)\right.}{\cos ^3(4 x)}\right)\left(\frac{\sin ^3(4 x)}{\left(\log _e(2 x+1)^5\right.}\right)\right)$ is equal to [JEE MAINS 2023]
1) $24$
2) $9$
3) $18$
4) $15$

Solution:

$
\begin{aligned}
& \lim _{x \rightarrow 0}\left[\frac{1-\cos ^2 3 x}{9 x^2}\right] \frac{9 x^2}{\cos ^3 4 x} \cdot \frac{\left(\frac{\sin 4 x}{4 x}\right)^3 \times 64 x^3}{\left[\frac{\ln (1+2 x)}{2 x}\right]^5 \times 32 x^5} \\
& \lim _{x \rightarrow 0} 2\left(\frac{1}{2} \times \frac{9}{1} \times \frac{1 \times 64}{1 \times 32}\right)=18
\end{aligned}
$
Hence, the answer is the option (3).

Example 3: Let $a_1, a_2, a_3, \ldots, a_n n$ be $n$ positive consecutive terms of an arithmetic progression. If this is its common difference, then: $\lim\limits _{n \rightarrow \infty} \sqrt{\frac{d}{n}}\left(\frac{1}{\sqrt{a_1}+\sqrt{a_2}}+\frac{1}{\sqrt{a_2}+\sqrt{a_3}}+\ldots \ldots+\frac{1}{\sqrt{a_{n-1}}+\sqrt{a_n}}\right)$ is

[JEE MAINS 2023]
1) $\frac{1}{\sqrt{d}}$
2) $1$
3) $\sqrt{d}$
4) $0$

Solution:

$
\begin{aligned}
& \lim\limits_{n \to \infty} \sqrt{\frac{d}{n}} \left( \frac{\sqrt{a_1} - \sqrt{a_2}}{a_1 - a_2} + \frac{\sqrt{a_2} - \sqrt{a_3}}{a_2 - a_3} + \cdots + \frac{\sqrt{a_{n-1}} - \sqrt{a_n}}{a_{n-1} - a_n} \right) \\
& = \lim\limits_{n \to \infty} \sqrt{\frac{d}{n}} \left( \frac{\sqrt{a_1} - \sqrt{a_2} + \sqrt{a_2} - \sqrt{a_3} + \cdots + \sqrt{a_{n-1}} - \sqrt{a_n}}{-d} \right) \\
& = \lim\limits_{n \to \infty} \sqrt{\frac{d}{n}} \left( \frac{\sqrt{a_n} - \sqrt{a_1}}{d} \right) \\
& = \lim\limits_{n \to \infty} \frac{1}{\sqrt{n}} \left( \frac{\sqrt{a_1 + (n - 1) d} - \sqrt{a_1}}{\sqrt{d}} \right) \\
& = \lim\limits_{n \to \infty} \frac{1}{\sqrt{d}} \left( \sqrt{\frac{a_1 + (n - 1) d}{n}} - \frac{\sqrt{a_1}}{n} \right) \\
& = 1
\end{aligned}
$

Hence, the answer is the option 2

Example 4: If $x$ approaches $2$, then the approximate value of is
1) $4$
2) $2$
3) $3$
4) $1$

Solution:

As we have learned

Condition on Limits -
The limit does not give actual value. It gives an approximate value.
- wherein
$f(x)=\frac{x^2+x-2}{x-1}$
$x$ is not defined at $\mathrm{x}=1$ but for $\mathrm{x}>1 \& \mathrm{x}<1$ it gives approximate values.

When x approaches $2, x-2$ simplifies to $\mathrm{x}+2$
Limit approaches to $4$
Hence, the answer is the option 1.

Example 5: If $x$ approaches $3$ , then $\frac{x^2-5 x+6}{x^2-4 x+3}$ has approximate value
1) $\frac{1}{2}$
2) $0$
3) $1$
4) $\frac{3}{2}$

Solution:

Condition on Limits -

The limit does not give actual value. It gives an approximate value.
- wherein

$
f(x)=\frac{x^2+x-2}{x-1}
$

$x$ is not defined at $x=1$ but for $x>1 \& x<1$ it gives approximate values.

$
\frac{x^2-5 x+6}{x^2-4 x+3}=\frac{(x-2)(x-3)}{(x-1)(x-3)}
$
When x approaches $3, \frac{x^2-5 x+6}{x^2-4 x+3}$ simplifies to $\frac{x-2}{x-1}$
$\therefore \frac{x-2}{x-1}$ approaches $\frac{3-2}{3-1}=1 / 2$
Hence, the answer is the option 1.

Summary

Limits are essential in studying how functions behave as their input approaches specific values. An understanding of limits is quite essential to be able to venture out into the study of the basic principles of calculus, where changes and revisions will be made for very different applications like Engineering, Physics, and Economics. They allow us to investigate functions near essential points, even those at which the functions are not defined, and they provide a view of continuity and behavior for derivatives and integrals.