Linear Differential Equation

Linear differential equations are the fundamentals that help us to understand how things change over time. These equations are used in various real-world situations, like calculating population growth, how currents flow in electrical circuits etc. The relationship between a function and its derivative, or rate of change is described by linear differential equations, where these relationships are linear, meaning they can be represented as straight lines when plotted in a graph.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. What is a Differential Equation?
2. What is a Linear Differential Equation?
3. Solve Linear Differential Equation using Integrating Factor
4. Solved Examples Based On Linear Differential Equation
5. Summary
6. Frequently Asked Questions (FAQs):

In this article, we will cover the concept of linear differential equations. This concept falls under the broader category of differential equations, which is a crucial chapter in class 12 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of eighty-two questions have been asked on this concept, including one in 2014, two in 2015, two in 2016, one in 2018, ten in 2019, five in 2020, twenty in 2021, twenty-one in 2022, and ninteen in 2023.

What is a Differential Equation?

A differential equation is an equation involving one or more terms and the derivatives of one dependent variable with respect to the other independent variable.

Differential equation: $\frac{d y}{d x} =f(x)$
Where " $x$ " is an independent variable and " $y$ " is a dependent variable

Example of differential equation: $x \frac{d y}{d x}+2 y=0$
The above-written equation involves variables as well as the derivative of the dependent variable $\mathrm{y}$ with respect to the independent variable $\mathrm{x}$. Therefore, it is a differential equation.
The following relations are some of the examples of differential equations:
(i) $\frac{d y}{d x}=\sin 2 x+\cos x$
(ii) $\mathrm{k} \frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}=\left[1+\left(\frac{\mathrm{dy}}{\mathrm{dx}}\right)^2\right]^{3 / 2}$

What is a Linear Differential Equation?

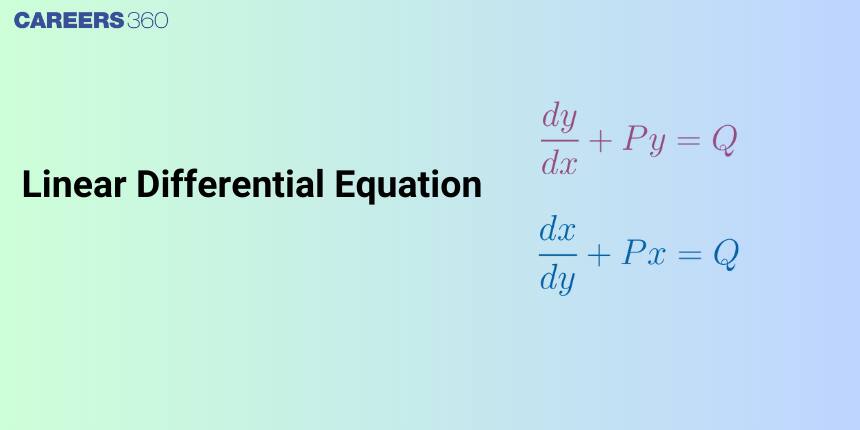
The linear differential equation is a linear equation that involves one or more terms consisting of derivatives of the dependent variable concerning one or more independent variables.

The general equation of the first-order differential equation is the form of

$
\frac{d y}{d x}+P(x) \cdot y=Q(x)
$
Where P(x) and Q(x) are functions of x only or constants.

Non-Linear Differential Equation

When the equation is not linear in an unknown function and its derivatives, then it is said to be a nonlinear differential equation. It provides diverse solutions which can be seen for chaos.

Solving Linear Differential Equations

To solve these types of equations, various methods can be used:

1. Separation of Variables: Applicable to those types of equations which is very simple in which variables can be separated easily on each side of the equation.
2. Integrating Factor: For first-order(degree) linear equations of the form dy/dx+P(x)y=Q(x), we multiply by an integrating factor to simplify.
3. Characteristic Equation: For constant coefficient linear differential equations, we solve the characteristic polynomial to find the solutions of the equations.

Solve Linear Differential Equation using Integrating Factor

Integrating factor: A term, which when multiplied by an expression, converts it to an exact differential i.e. a function which is the derivative of another function.

We have, $\frac{d y}{d x}+P(x) \cdot y=Q(x)$
multiply both sides of Eq (i) by $\int e^{P(x) d x}$, we get
i.e. $\quad e^{\int P(x) d x} \cdot \frac{d y}{d x}+y \cdot P(x) \frac{d}{d x}\left(e^{\int P(x) d x}\right)=Q e^{\int P(x) d x}$
or $\quad \frac{d}{d x}\left(\mathrm{ye}^{\int P(x) d x}\right)=\mathrm{e}^{\int P(x) d x} \cdot Q(x)$
Integrating both sides, we get
or $\quad \int \mathrm{d}\left(y e^{\int P(x) d x}\right)=\int\left(e^{\int P(x) d x} \cdot Q(x)\right) d x$
$\Rightarrow \quad y \mathrm{e}^{\int P(x) d x}=\int \mathrm{Q}(\mathrm{x}) \mathrm{e}^{\int \mathrm{P}(\mathrm{x}) d \mathrm{dx}} \mathrm{dx}+\mathrm{C}$

Which is the required solution of the given differential equation.

The term $\mathrm{e}^{/ \mathrm{P}(\mathrm{x}) \mathrm{dx}}$ which convert the left hand expression of the equatio into a perfect differential is called an Integrating factor (IF).

Thus, we remember the solution of the above equation as

$
y(\mathrm{IF})=\int Q(\mathrm{IF}) d x+C
$
Note : Sometimes a given differential equation can be made linear if we take x as the dependent variable and y as the independent variable. So, we can check the equation with respect to both x and y.

Recommended Video Based on Linear Differential Equation

Solved Examples Based On Linear Differential Equation

Example 1: If the solution curve of the differential equation
$\left(y-2 \log _e x\right) d x+\left(x \log _e x^2\right) d y=0, x>1_{\text {passes through the points }}\left(e, \frac{4}{3}\right)$ and $\left(e^4, \alpha\right)$, then $\alpha$ is equal to $\qquad$
[JEE Main 2023]
Solution:

$
\begin{aligned}
& (y-2 \ln x) d x+(2 x \ln x) d y=0 \\
& d y(2 x \ln x)=[(2 \ln x)-y] d x \\
& \frac{d y}{d x}=\frac{1}{x}-\frac{y}{2 x \ln x} \\
& \frac{\mathrm{dy}}{\mathrm{dx}}+\frac{\mathrm{y}}{2 \mathrm{x} \ln \mathrm{x}}=\frac{1}{\mathrm{x}} \\
& \mathrm{I} . \mathrm{F}=e^{\int \frac{1}{2 x \ln x} d x} \\
& =\mathrm{e}^{\frac{1}{2} \int \frac{\mathrm{dt}}{\mathrm{t}}}=\mathrm{e}^{\frac{1}{2} \ln (\ln x)} \\
& \Rightarrow \mathrm{I} \cdot \mathrm{F}=(\ln x)^{1 / 2} \\
& \therefore \mathrm{y} \sqrt{\ln \mathrm{x}}=\int \frac{\sqrt{\ln \mathrm{x}}}{\mathrm{x}} \mathrm{dx} \quad\left(\text { Let }, \ln \mathrm{x}=\mathrm{u}^2\right) \\
& =2 \int u^2 d u
\end{aligned}
$

$
\begin{aligned}
& (y-2 \ln x) d x+(2 x \ln x) d y=0 \\
& d y(2 x \ln x)=[(2 \ln x)-y] d x \\
& \frac{d y}{d x}=\frac{1}{x}-\frac{y}{2 x \ln x} \\
& \frac{\mathrm{dy}}{\mathrm{dx}}+\frac{\mathrm{y}}{2 \mathrm{x} \ln \mathrm{x}}=\frac{1}{\mathrm{x}} \\
& \mathrm{I} . \mathrm{F}=e^{\int \frac{1}{2 x \ln x} d x} \\
& =\mathrm{e}^{\frac{1}{2} \int \frac{\mathrm{dt}}{\mathrm{t}}}=\mathrm{e}^{\frac{1}{2} \ln (\ln x)} \\
& \Rightarrow \mathrm{I} \cdot \mathrm{F}=(\ln x)^{1 / 2} \\
& \therefore \mathrm{y} \sqrt{\ln \mathrm{x}}=\int \frac{\sqrt{\ln \mathrm{x}}}{\mathrm{x}} \mathrm{dx} \quad\left(\text { Let }, \ln \mathrm{x}=\mathrm{u}^2\right) \quad \frac{1}{x} d x=2 \mathrm{udu} \\
& =2 \int u^2 d u
\end{aligned}
$

$
\begin{aligned}
& \mathrm{y} \sqrt{\ln x}=\frac{2}{3}(\ln x)^{3 / 2}+c \leftarrow\left(e, \frac{4}{3}\right) \\
& \frac{4}{3}=\frac{2}{3}+c \Rightarrow c=\frac{2}{3} \\
& y \sqrt{\ln x}=\frac{2}{3}(\ln x)^{3 / 2}+\frac{2}{3} \leftarrow\left(e^4, \alpha\right) \\
& \alpha \cdot 2=\frac{2}{3} \times 8+\frac{2}{3} \\
& \alpha=3
\end{aligned}
$

Hence, the answer is 3 .
Example 2: If $y=y(x)$ is the solution of the differential equation $\frac{d y}{d x}+\frac{4 x}{\left(x^2-1\right)} y=\frac{x+2}{\left(x^2-1\right)^{\frac{5}{2}}}, x>1 \quad$ such that $y(2)=\frac{2}{9} \log _c(2+\sqrt{3})$ and $y(\sqrt{2})=\alpha \log _{\mathrm{c}}(\sqrt{\alpha}+\beta)+\beta-\sqrt{\gamma}, \alpha, \beta, \gamma, \in \mathrm{N}$, then $\alpha \beta \gamma$ is equal to $\qquad$ .
[JEE Main 2023]
Solution:
Given differential equation $\frac{d y}{d x}+\frac{4 x}{\left(x^2-1\right)} y=\frac{x+2}{\left(x^2-1\right)^{5 / 2}}$ is linear D.E.

$
\begin{aligned}
& \text { I.F. }=\int_e \frac{4 x}{x^2-1} d x=e_e 2 \ln \left(x^2-1\right)={ }_e \ln \left(x^2-1\right)^2=\left(x^2-1\right)^2 \\
& y\left(x^2-1\right)^2=\int \frac{x+2}{\left(x^2-1\right)^{5 / 2}}\left(x^2-1\right)^2 d x \\
& =\int \frac{x}{\sqrt{x^2-1}} d x+\int \frac{2 d x}{\sqrt{x^2-1}} \\
& =\sqrt{x^2-1}+2 \ln \left[x+\sqrt{x^2-1}\right]+C \\
& \text { put } y(2)=\frac{2}{9} \ln (2+\sqrt{3}) \\
& \frac{2}{9} \ln (2+\sqrt{3})(9)=\sqrt{3}+2 \ln [2+\sqrt{3}]+C \\
& =C=-\sqrt{3} \\
& \text { put } x=\sqrt{2} \\
& y=1+2 \ln [\sqrt{2}+1]-\sqrt{3}
\end{aligned}
$

$
\begin{aligned}
& \frac{2}{9} \ln (2+\sqrt{3})(9)=\sqrt{3}+2 \ln [2+\sqrt{3}]+C \\
& =C=-\sqrt{3} \\
& \text { put } x=\sqrt{2} \\
& y=1+2 \ln [\sqrt{2}+1]-\sqrt{3} \\
& \alpha=2, \beta=1=\gamma=3 \\
& \alpha \beta \gamma=2(1)(3)=6
\end{aligned}
$

Hence, the answer is 6 .
Example 3: If $y=y(x)$ is the solution curve of the differential equation $\frac{d y}{d x}+y \tan x=x \sec x, 0 \leq x \leq \frac{\pi}{3}, y(0)=1$, then $y\left(\frac{\pi}{6}\right)$ is equal to [JEE Main 2023]

Solution:
Given D.E. is linear D.E.

$
\begin{aligned}
& \text { I.F. }=e^{\int \tan x d x} \\
& =e^{\ell \operatorname{nsec} x}=\operatorname{secx} \\
& y \sec x=\int x \sec ^2 x d x \\
& =\mathrm{x} \tan \mathrm{x}-\int \tan x d x \\
& \Rightarrow \quad y \sec x=x \tan x-\ell \mathrm{n} \operatorname{sect}+c \\
& \text { Put } y(0)=1 \\
& 1=0-0+\mathrm{c} \Rightarrow \mathrm{c}=1 \\
& \mathrm{Y}(\mathrm{x})=\frac{x \tan x}{\sec x}-\frac{\ell \operatorname{nsec} x}{\sec x}+\frac{1}{\sec x} \\
& y\left(\frac{\pi}{6}\right)=\frac{\left(\frac{\pi}{6}\right)\left(\frac{1}{\sqrt{3}}\right)}{\left(\frac{2}{\sqrt{3}}\right)}-\frac{\ln \left(\frac{2}{\sqrt{3}}\right)}{\left(\frac{2}{\sqrt{3}}\right)}+\frac{\sqrt{3}}{2} \\
& =\frac{\pi}{12}-\frac{\sqrt{3}}{2} \ell n\left(\frac{2}{\sqrt{3}}\right)+\frac{\sqrt{3}}{2} \ell n e \\
& =\frac{\pi}{12}-\frac{\sqrt{3}}{2} \ell n\left(\frac{2}{e \sqrt{3}}\right)
\end{aligned}
$

Hence, the required answer is $\frac{\pi}{12}-\frac{\sqrt{3}}{2} \log _e\left(\frac{2}{e \sqrt{3}}\right)$

Example 4: Let $\mathrm{y}=\mathrm{y}(\mathrm{x})$ be the solution of the differential equation
$x\left(1-x^2\right) \frac{d y}{d x}+\left(3 x^2 y-y-4 x^3\right)=0, x>1$, with $y(2)=-2$. Then $y(3)$ equal to
[JEE Main 2022] is

Solution:

$
\begin{aligned}
& \frac{d y}{d x}+\frac{\left(3 x^2-1\right)}{x\left(1-x^2\right)} y=\frac{4 x^3}{x\left(1-x^2\right)} \\
& \text { I.F }=e^{\int\left(\frac{x^2-1}{x\left(1-x^2\right)}+\frac{2 x^2}{x\left(1-x^2\right)}\right) d x}=e^{-\ln x-\ln \left(1-x^2\right)}=\frac{1}{x\left(1-x^2\right)} \\
& \Rightarrow y \times \frac{1}{x\left(1-x^2\right)}=\int \frac{4 x^3}{x^2\left(1-x^2\right)^2} d x=\frac{+2}{1-x^2}+c \\
& \text { at } x=2, y=-2 \Rightarrow c=1 \\
& \Rightarrow y=2 x+x\left(1-x^2\right) \\
& \text { at } x=3 ; y=6+3(1-9)=-18
\end{aligned}
$

Hence, the required answer is -18 .
Example 5: Let $\mathrm{y}=\mathrm{y}(\mathrm{x})$ be the solution curve of the differential equation

$
\begin{aligned}
& \frac{d y}{d x}+\frac{1}{x^2-1} y=\left(\frac{x-1}{x+1}\right)^{1 / 2}, x>1 \\
& \left(2, \sqrt{\frac{1}{3}}\right) . \text { Then } \sqrt{7} y(8) \\
& \text { is equal to: } \\
& \text { [JEE Main 2022] }
\end{aligned}
$

Solution:

$
\begin{aligned}
& \text { For } I \cdot F \\
& =\int \frac{1}{(x-1)(x+1)} d x \\
& =\frac{1}{2}\left[\int \frac{1}{x-1} d x-\int \frac{1}{x+1} d x\right] \\
& =\frac{1}{2} \ln \left(\frac{x-1}{x+1}\right) \\
& =\ln \sqrt{\frac{x-1}{x+1}} \\
& \therefore I \cdot F \cdot=\sqrt{\frac{x-1}{x+1}}
\end{aligned}
$

$\therefore$ Solution is

$
\begin{aligned}
& y \cdot \sqrt{\frac{x-1}{x+1}}=\int \frac{x-1}{x+1} d x \\
& y \sqrt{\frac{x-1}{x+1}}=x-2 \ln (x+1)+c
\end{aligned}
$

Using $\left(2, \sqrt{\frac{1}{3}}\right)$

$
\begin{aligned}
& \sqrt{\frac{1}{3}} \cdot \sqrt{\frac{1}{3}}=2-2 \ln 3+c \\
& c=2 \ln 3-\frac{5}{3} \\
& \text { put } x=8 \\
& y \sqrt{\frac{7}{9}}=8-2 \ln 9+2 \ln 3-\frac{5}{3} \\
& \sqrt{7} y=3\left[\frac{19}{3}-2 \ln 3\right] \\
& =19-6 \ln 3
\end{aligned}
$

Hence, the required answer is $19-6 \ln 3$

Summary

Differential equations are powerful tools for modeling and understanding the behavior of real-world dynamic systems. By translating real- life events into differential equations, we can predict future behavior and gain insight into the underlying mechanisms. The example of the increasing population shows that a simple differential equation can explain a complex world phenomenon.Linear differential equations are powerful tools or technology for modeling and solving problems in various fields. Understanding their formulation and methods of solution is essential for anyone working in science, engineering, economics, and beyond.

Frequently Asked Questions (FAQs):

1. What is a linear differential equation?

Ans. Linear differential equations are those types of equations in which the variable and its derivative occur only in the first degree.

2. What is an example of a linear differential equation?

Ans. Examples of linear differential equations are:

x dy/dx+3y = x2

dx/dy – x/y = 4y

dy/dx + y tan x = 5x2

3. What is the difference between linear and nonlinear equations?

Ans. A linear equation will always exist for all values of x and y but nonlinear equations may or may not have solutions for all values of x and y.

4. What is the Integrating Factor?

Ans. An integrating factor is a function that is selected in order to solve the given differential equation. If the differential equation is of the form dy/dx + Q(x)y = R(x) then the integrating factor is given by Integrating Factor(I.F) = e∫R dx.

5. How to solve the first-order differential equation?

Ans.

Step1- First write the equation in the form of dy/dx+Py = Q, where P and Q are constants of x only

Step2-Find integrating factor, IF = e∫Pdx

Step3- write the solution in the form of y (I.F) = ∫Q × I.F