Multiplication of Complex Numbers
A complex number is written in the form $a + bi$, where $i = \sqrt{-1}$. Multiplying two complex numbers follows a simple formula that expands just like algebraic expressions, but with the special rule of powers of $i$. This concept is widely used in solving equations, trigonometry, and advanced problems in mathematics. In this article, we will learn the formula, rules, properties, and solved examples of multiplication of complex numbers in detail.
This Story also Contains
- Introduction to Complex Numbers
- Multiplication of Complex Numbers
- Properties Of Multiplication Of Complex Numbers
- Rules and Formula for Multiplication of Complex Numbers
- Multiplication of Complex Numbers in Different Forms
- Solved Examples based on Multiplication of Complex Numbers
- List of topics related to Multiplication Of Complex Numbers
- NCERT Resources
- Practice Questions based on Multiplication Of Complex Numbers
Introduction to Complex Numbers
The numbers which have no real meaning in the real number system are represented in complex form. The general form of a complex number is $a + ib$, where $i$ is iota or $i = \sqrt{-1}$.
A number of the form $a + ib$ is called a complex number, where $a$ and $b$ are real numbers and $i$ is iota. We usually denote a complex number by the letters $z, z_1, z_2$, etc.
For example, $z = 5 + 2i$ is a complex number.
$5$ is called the real part and is denoted by $\text{Re}(z)$.
$2$ is called the imaginary part and is denoted by $\text{Im}(z)$.
Basic Form of a Complex Number ($a + bi$)
A complex number is written in the basic form $a + bi$, where $a$ is the real part and $b$ is the imaginary part. Here, $i = \sqrt{-1}$ is known as iota. For example, in $z = 7 + 3i$, we have $\text{Re}(z) = 7$ and $\text{Im}(z) = 3$. This standard form is the foundation for addition, subtraction, and multiplication of complex numbers.
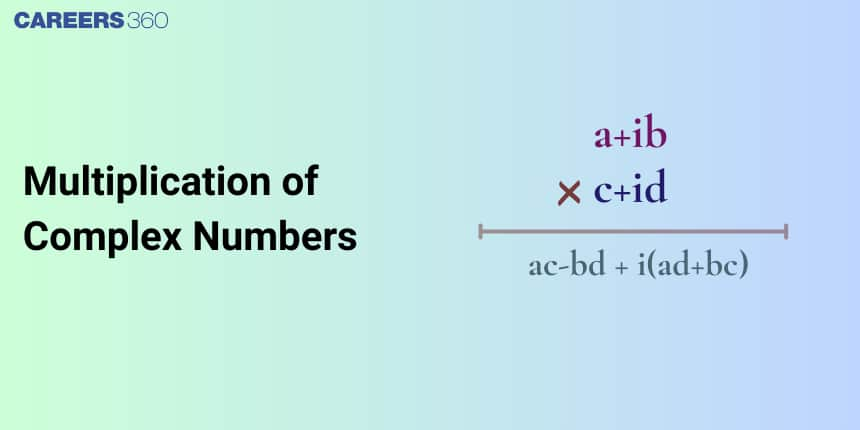
Multiplication of Complex Numbers
The multiplication of complex numbers is a key concept in mathematics and forms an important part of Class 11 Maths and competitive exams. It follows a simple formula similar to binomial expansion, with the special rule $i^2 = -1$. Learning the rules, properties, and solved examples of multiplying two complex numbers helps students simplify problems quickly and accurately.
Formula for Multiplication
Multiplying two complex numbers is similar to multiplying two binomials.
Let $z_1 = a + ib$ and $z_2 = c + id$ be any two complex numbers. Then, their multiplication is:
$\begin{aligned} z_1 \cdot z_2 & = (a + ib)(c + id) \\ & = ac + iad + ibc + i^2bd \\ & = ac + i(ad + bc) - bd \\ & = (ac - bd) + i(ad + bc) \end{aligned}$
Thus, the formula for multiplication is:
$(a+ib)(c+id) = (ac - bd) + i(ad + bc)$
Example of Multiplication
Let $z_1 = (4 + 3i)$ and $z_2 = (2 - 5i)$. Then,
$\begin{aligned} (4 + 3i)(2 - 5i) & = 4(2) - 4(5i) + 3i(2) - (3i)(5i) \\ & = 8 - 20i + 6i - 15(i^2) \\ & = (8 + 15) + (-20 + 6)i \\ & = 23 - 14i \end{aligned}$
So, $z_1 \cdot z_2 = 23 - 14i$.
Properties Of Multiplication Of Complex Numbers
Name of the Property | Description | Expression |
Closure property | The product of any two complex numbers is always a complex number only | $z_1 \cdot z_2=z$ |
Commutative property | Change of any order of any complex numbers does not change the result of their product (L.H.S=R.H.S) | $z_1 \cdot z_2=z_2 \cdot z_1$ |
Associative property | Regrouping of any complex numbers does not change the result of their product (L.H.S=R.H.S) | $\begin{aligned} & \left(z_1 \cdot z_2\right) \cdot z_3= z_1 \cdot\left(z_2 \cdot z_3\right)\end{aligned}$ |
Distributive property | Multiplying any complex number with the sum of two complex numbers is given by: | $\begin{aligned} & z_1 \cdot\left(z_2+z_3\right)=z_1 \cdot z_2+z_1 \cdot z_3\end{aligned}$ |
Multiplicative identity: if the multiplication of a complex number $z_1$ with another complex number $z_2$ is $z_1$, then $z_2$ is called the multiplicative identity. We have $z \times 1 = z = 1 \times z$, so 1 is the multiplicative identity.
Multiplicative inverse: For every non-zero complex $z=a+i b,(a \neq 0, b \neq 0)$ we have the complex number $\frac{a}{a^2+b^2}+i \frac{-b}{a^2+b^2}\left(\right.$ denoted by $\frac{1}{z}$ or $\left.z^{-1}\right)$ called the multiplicative inverse of z.
Rules and Formula for Multiplication of Complex Numbers
The rules and formula for multiplication of complex numbers make it easy to expand and simplify expressions involving $a+bi$ and $c+di$. By applying the distributive law and using the identity $i^2 = -1$, the product of two complex numbers is reduced to a simple standard form. We have discussed about the rules and formulae below in detail:
Standard Formula $(a+bi)(c+di) = (ac - bd) + (ad + bc)i$
When two complex numbers $z_1 = a + bi$ and $z_2 = c + di$ are multiplied, the result follows the formula:
$(a+bi)(c+di) = (ac - bd) + (ad + bc)i$
This formula is similar to expanding binomials in algebra but uses the special property $i^2 = -1$.
Step-by-Step Method to Multiply Two Complex Numbers
To multiply complex numbers correctly, follow these steps:
Write both numbers in the form $a + bi$ and $c + di$.
Expand using distributive law: $(a+bi)(c+di)$.
Simplify using $i^2 = -1$.
Combine the real and imaginary parts to get the result.
Example: Multiply $z_1 = 3 + 2i$ and $z_2 = 1 - 4i$.
$(3+2i)(1-4i) = (3)(1) + (3)(-4i) + (2i)(1) + (2i)(-4i)$
$= 3 - 12i + 2i - 8(i^2)$
$= 3 - 10i + 8$
$= 11 - 10i$
So, $z_1 \cdot z_2 = 11 - 10i$
Multiplication of Complex Numbers in Different Forms
The multiplication of complex numbers in different forms can be done using the standard form $a+bi$, the polar form $r(\cos\theta+i\sin\theta)$, or Euler’s form $re^{i\theta}$. Each method has its own advantage, and mastering them helps in solving higher-level problems in trigonometry, calculus, and competitive exams.
Multiplication in Standard Form ($a+bi$)
In standard form, multiplication directly applies the formula: $(a+bi)(c+di) = (ac - bd) + (ad + bc)i$
This is the most common method taught in Class 11 Maths NCERT and used in competitive exams.
Multiplication in Polar Form ($r(\cos\theta + i\sin\theta)$)
If a complex number is written as $z = r(\cos\theta + i\sin\theta)$, then the product of two complex numbers is: $z_1 \cdot z_2 = r_1r_2 \big(\cos(\theta_1 + \theta_2) + i\sin(\theta_1 + \theta_2)\big)$
This rule is known as De Moivre’s Theorem and is useful for trigonometry and advanced problems.
Multiplication Using Euler’s Formula ($re^{i\theta}$)
In exponential form, a complex number is expressed as $z = re^{i\theta}$. The multiplication rule becomes:
$z_1 \cdot z_2 = r_1r_2 \, e^{i(\theta_1 + \theta_2)}$
This method is very efficient in higher-level mathematics, especially in engineering and physics.
Solved Examples based on Multiplication of Complex Numbers
Example 1: The multiplicative inverse of the complex number $z=\frac{5+i \sqrt{2}}{1-i \sqrt{2}}$ is:
Solution:
As we have learnt, the multiplicative inverse of $z$ is $\frac{1}{z}$
Now, $z = \frac{5+i \sqrt{2}}{1-i \sqrt{2}}$
$\frac{1}{z} = \frac{1-i \sqrt{2}}{5+i \sqrt{2}}$
$= \frac{1-i \sqrt{2}}{5+i \sqrt{2}} \cdot \frac{5-i \sqrt{2}}{5-i \sqrt{2}}$
$= \frac{5-i \sqrt{2}-i 5 \sqrt{2}-2}{25+2}$
$= \frac{3-6 \sqrt{2} i}{27}$
$= \frac{3(1-2 \sqrt{2} i)}{27}$
$= \frac{1-2 \sqrt{2} i}{9}$
So, the multiplicative inverse is $\frac{1-2 i \sqrt{2}}{9}$.
Hence, the answer is option 2.
Example 2: Let $z_1=2+3 i$ and $z_2=-1-5 i$ then $z_1 z_2$ equals
Solution:
Multiplication of Complex Numbers -
$(a+ib)(c+id)=(ac-bd)+i(bc+ad)$
$z_1 z_2=(2+3 i)(-1-5 i)=\{(2)(-1)-(3)(-5)\}+i\{(3)(-1)+(2)(-5)\}$
$\Rightarrow z_1 z_2=13-13 i$
Hence, the answer is 13-13i
Example 3: For two non-zero complex numbers $z_1$ and $z_2$ if $\operatorname{Re}\left(z_1 z_2\right)=0$ and $\operatorname{Re}\left(z_1+z_2\right)=0$, then which of the following are possible?
A. $\operatorname{lm}\left(z_1\right)>0$ and $\operatorname{Im}\left(z_2\right)>0$
B. $\operatorname{lm}\left(z_1\right)<0$ and $\operatorname{Im}\left(z_2\right)>0$
C. $\operatorname{lm}\left(z_1\right)>0$ and $\operatorname{Im}\left(z_2\right)<0$
D. $\operatorname{lm}\left(z_1\right)<0$ and $\operatorname{Im}\left(z_2\right)<0$
Choose the correct answer from the options given below:
1) $B$ and $D$
2) A and B
3) $B$ and $C$
4) A and C
Solution:
$\operatorname{Re}\left(z_1 z_2\right)=0$ and $\operatorname{Re}\left(z_1+z_2\right)=0$
let $z_1=a_1+i h_1 a_{n t} z_n=a_2+i_2$
$a_1 z_2=\left(a_1 a_2-b_1 b_2\right)+i\left(a_1 b_2+b_1 a_2\right)$
$\because R e\left(z_1 z_2\right)=a_1 a_2-b_1 b_2=0$
$\therefore a_1 a_2=b_1 b_2 \ldots \ldots \ldots(1)$
and $\operatorname{Re}\left(z_1+z_2\right)=0 \Rightarrow a_1+a_2=0$
$E a_2=-a_1 \ldots \ldots \ldots \ldots \ldots(2)$
rom (1) and (2)
$-b_1 b_2=-1_1^2<1$
The product of $b_1 b_2$ is a Negative
- Im (z) and Im (z) are also of opposite sign
Hence, the answer is the option 3.
List of topics related to Multiplication Of Complex Numbers
Below is a list of topics related to multiplication of complex numbers, covering rules, formulas, solved examples, and important properties. These topics help students clearly understand how to multiply complex numbers and simplify results using the powers of $i$.
NCERT Resources
Below are the NCERT resources for Class 11 Maths Chapter 5 – Complex Numbers and Quadratic Equations, including solutions, exemplar questions, and notes. These resources make it easier to learn concepts like powers of iota, properties of complex numbers, and quadratic equations with clear step-by-step methods.
NCERT Solutions for Class 11 Maths Chapter 5 -Complex Numbers and Quadratic Equations
NCERT Exemplar Class 11 Maths Solutions for Chapter 5 - Complex Numbers and Quadratic Equations
NCERT Class 11 Maths Notes for Chapter 5 - Complex Numbers and Quadratic Equations
Practice Questions based on Multiplication Of Complex Numbers
Below are some practice questions based on multiplication of complex numbers that help students master the application of formulas and rules. These problems strengthen the understanding of how to multiply two complex numbers and simplify results using the powers of $i$.
Multiplication Of Complex Numbers - Practice Question MCQ
We have shared practice questions sets for related topics below:
Frequently Asked Questions (FAQs)
Multiplication of two complex numbers means the multiplication of one number to another i.e. $z_1z_2$.
The multiplication inverse of z is 1/z.
Suppose $z 1=\mathrm{a}+\mathrm{ib}$ and $z^2=\mathrm{c}+$ idare two complex numbers, then the multiplication or product of these two complex numbers can be calculated using the formula $z 122=(a c-d b)+i(a d+b c)$
Yes, complex numbers are closed under multiplication.
The following properties can be defined for the multiplication of complex numbers:
Closure law
Commutative law
Associative law
Distributive law
The existence of multiplicative identity
The existence of a multiplicative inverse