Powers of Iota

The term iota represented by i which is widely used in complex numbers. It is represented by $\sqrt{-1}$ or $i$. In real life, this can be helped in solving some challenging problems of complex numbers. Iota is an imaginary unit number to express complex numbers, where i is defined as imaginary or unit imaginary. The value of the imaginary unit number, i is generated, when there is a negative number inside the square root. Thus, the value of i is given as $\sqrt{-1}$.

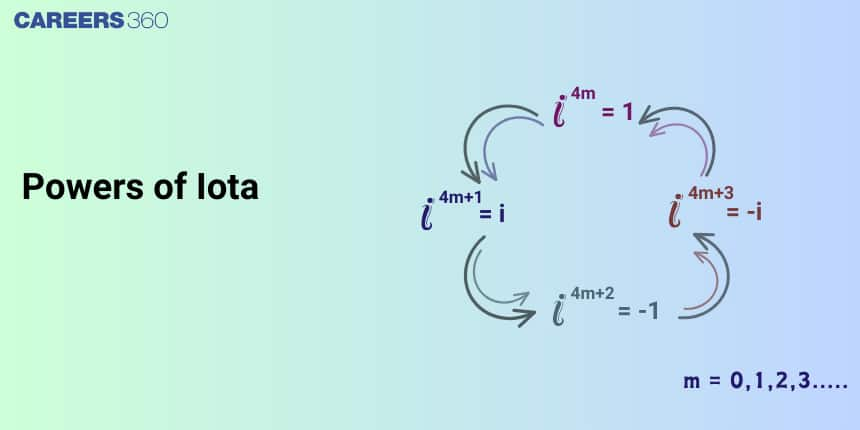
In this article, we will cover the concept of iota and its behavior by increasing its power. This concept falls under the broader category of complex numbers. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination (JEE Main), and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main exam (from 2013 to 2023), a total of two questions have been asked on this concept, including one in 2019, and one in 2020.

What is iota?

Iota is an imaginary unit number that is denoted by i and the value of iota is $\sqrt{-1} \text { i.e., } i=\sqrt{ -1}$. When we solve quadratic equations, we might have come across situations where the discriminant is negative. For example, consider the quadratic equation $x^2+x+1=0$. If we use the quadratic formula to solve this, we get the discriminant (the part inside the square root) as a negative value. Due to this the term iota is introduced.

For analyzing the powers of iota, we calculate the first few powers of iota. i.e $i=\sqrt{-1}$, $i^2=-1$, $i^3=-\sqrt{-1}$, $i^4=1$

Value of iota ($i$)

The square of any real number, whether it is positive or negative or zero is always non-negative, i.e. $x^2 \geq 0$ for all x ∈ R.

Hence, the equation $x^2+x+1=0$ is not satisfied for any real value of x or not solvable in a real number system.

Thus, the equation $x^2+x+1=0$ has an imaginary solution. ‘Eular’ was the first mathematician to introduce the symbol i (read as ‘iota’). The imaginary number i is defined as the square root of −1.

Hence, the equation
$
\begin{aligned}
& x^2+1=0 \\
& \Rightarrow \quad x^2=-1
\end{aligned}
$
$
\text { or, } \quad \mathrm{x}= \pm \sqrt{-1}= \pm i
$
Equation, $\mathrm{x}^2+1=0$ has two solution, $\mathrm{x}=i$ and $\mathrm{x}=-i$.

$
\begin{aligned}
\sqrt{-1} & =\mathrm{i} \\
1^2 & =(\sqrt{-1})^2=-1
\end{aligned}
$
We can write the square root of any negative number as a multiple of i. Consider the square root of -25

$
\begin{aligned}
\sqrt{-25} & =\sqrt{25(-1)} \\
& =\sqrt{25} \sqrt{-1} \\
& =5 \mathrm{i}
\end{aligned}
$

Value of power of iota

1) If the power of iota is the whole number

$
\begin{aligned}
& \mathrm{i}^0=1, \mathrm{i}^1=\mathrm{i}, \mathrm{i}^2=(\sqrt{-1})^2=-1 \\
& \mathrm{i}^3=\mathrm{i} \cdot \mathrm{i}^2=\mathrm{i} \times-1=-\mathrm{i} \\
& \mathrm{i}^4=\mathrm{i}^2 \cdot \mathrm{i}^2=-1 \times-1=1 \\
& \mathrm{i}^5=\mathrm{i} \cdot \mathrm{i}^4=\mathrm{i} \times 1=\mathrm{i}
\end{aligned}
$

In general,
$
i^{4 \mathbf{n}}=1, \quad i^{4 \mathbf{n}+\mathbf{1}}=i, \quad i^{4 \mathrm{n}+2}=-1, \quad i^{4 \mathrm{n}+\mathbf{3}}=-1
$

2) If the power of iota is the negative integer

\begin{aligned}

$\begin{aligned} & i^{-1}=\frac{1}{i}=\frac{i}{i^2}=\frac{i}{-1}=-i \\ & i^{-2}=\frac{1}{i^2}=-1 \\ & i^{-3}=\frac{1}{i^3}=\frac{i}{i^4}=i \\ & i^{-4}=\frac{1}{i^4}=\frac{1}{1}=1\end{aligned}$

The sum of four consecutive powers of iota (i) is zero

$
\begin{aligned}
& \mathrm{n} \in \mathbb{I} \text { and } \mathrm{i}=\sqrt{-1} \text {, then } \\
& \begin{aligned}
i^{\mathrm{n}}+i^{\mathrm{n}+1}+i^{\mathrm{n}+2}+i^{\mathrm{n}+3} & =i^{\mathrm{n}}\left(1+i+i^2+i^3\right) \\
& =i(1+i-1-i)=0
\end{aligned}
\end{aligned}
$

Solved Examples Based on Powers of Iota

Example 1: If $a<0$ and $b<0$, then $\sqrt{a }\sqrt{ b}$ is equal to:

Solution

Here, $a$ and $b$ are both negative

$
\begin{aligned}
& \sqrt{-a} \sqrt{-b}=\sqrt{(-1) a} \sqrt{(-1) b} \\
& \Rightarrow \sqrt{-1} \sqrt{a} \sqrt{-1} \sqrt{b}=i^2 \sqrt{a} \sqrt{b} \\
& \Rightarrow-1 \sqrt{|a||b|}
\end{aligned}
$
so the value is $-\sqrt{(|a||b|)}$
Hence, the answer is $-\sqrt{(|a||b|) }$.

Example 2: $(1+i)^8+(1-i)^9$ equals:

Solution:

$
(1+i)^8=\left((1+i)^2\right)^4=\left(1+i^2+2 i\right)^4=(1-1+2 i)^4=(2 i)^4=2^4 \cdot i^4=16
$
and
$
\begin{aligned}
& (1-i)^9=\left((1-i)^2\right)^4 \cdot(1-i)^1=\left(1+i^2-2 i\right)^4 \cdot(1-i) \\
& =(-2 i)^4(1-i)=(-2)^4 \cdot i^4 \cdot(1-i)=16(1-i)=16-16 i \\
& \therefore(1+i)^8+(1-i)^9=16+16-16 i=32-16 i
\end{aligned}
$

Hence, the answer is $32-16 i$

Example 3: The value of $i^{-99}$ is:

Solution:

$i^{-99}=\frac{1}{i^{99}}=\frac{i}{i^{100}}=i \quad\left(\because i^{100}=\left(i^4\right)^{25}=1\right)$

So the value is $i$

Hence, the answer is $i$.

Example 4: If $\left(\frac{1+i}{1-i}\right)^x=1$, then $x=$ ?

Solution:

Power of i in Complex Numbers -

$i^{4 n}=1, i^{4 n+1}=i, i^{4 n+2}=-1, i^{4 n+3}=-i$, where $n \in$ Integer
Now,
$
\begin{aligned}
& \left(\frac{1+i}{1-i}\right)^x=\left[\frac{(1+i)(1+i)}{(1-i)(1+i)}\right]^x=\left[\frac{(1+i)(1+i)}{2}\right]^x \\
& =\left[\frac{(1+i)^2}{2}\right]^x=\left[\frac{1-1+2 i}{2}\right]^x=(i)^x
\end{aligned}
$

And given that
$
\begin{aligned}
& \left(\frac{1+i}{1-i}\right)^x=1 \\
& i^x=(i)^{4 n} \\
& x=4 n
\end{aligned}
$

Hence, the answer is $4n$

Summary

Iota is a powerful term for complex numbers. It helps in solving complex number problems, AC circuit analysis in electrical engineering, quantum mechanics, and signal processing. The imaginary unit iii is fundamental in complex number theory, and its powers follow a predictable cycle. Understanding the behaviour of $i$ and its powers is essential for simplifying complex expressions and solving equations involving complex numbers.