Sandwich Theorem

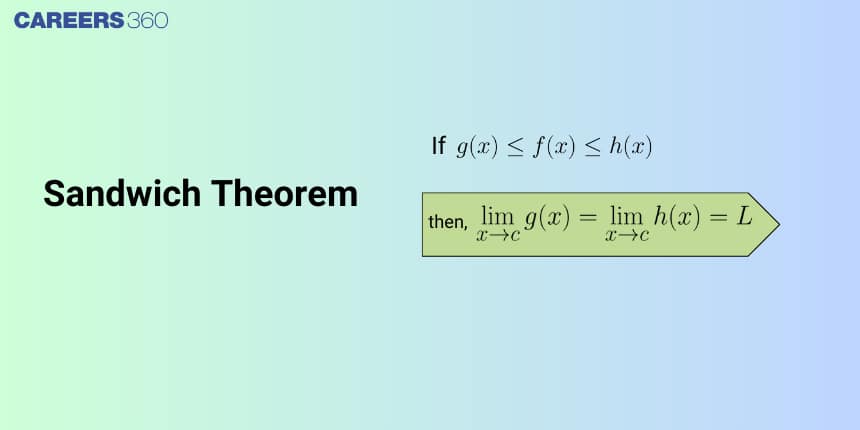
Sandwich Theorem of Limits

The Sandwich Theorem, also called the Squeeze Theorem, is a fundamental concept in calculus used to evaluate the limit of a function. It works by comparing a function with two other functions whose limits are known or easier to calculate.

Mathematically, let $f(x)$, $g(x)$, and $h(x)$ be real functions such that:

$f(x) \le g(x) \le h(x)$

for all $x$ in a neighbourhood of $x = a$. If

$\lim_{x \to a} f(x) = L = \lim_{x \to a} h(x)$,

then it follows that

$\lim_{x \to a} g(x) = L$.

This theorem states that if a function is “sandwiched” between two functions that have the same limit at a point, the middle function also approaches that limit. The domain of $g(x)$ must satisfy:

$f(x) \le g(x) \le h(x) \quad \text{for all } x \text{ in the domain of definition.}$

Floor Function (Greatest Integer Function)

Sometimes, the Floor Function is used in limit problems. The floor function, denoted as $[P]$, rounds a real number down to the nearest integer less than or equal to that number. For example:

$[3.7] = 3$ and $[-2.3] = -3$.

Proof of the Sandwich Theorem

Consider three real-valued functions $g(x)$, $f(x)$, and $h(x)$ such that:

$g(x) \le f(x) \le h(x)$

and

$\lim_{x \to a} g(x) = \lim_{x \to a} h(x) = L$.

By the definition of limits:

1. For $g(x)$, $\lim_{x \to a} g(x) = L$ implies that for every $\epsilon > 0$, there exists $\delta_1 > 0$ such that:

$|x - a| < \delta_1 \implies |g(x) - L| < \epsilon \quad \Rightarrow -\epsilon < g(x) - L < \epsilon \quad (i)$

2. For $h(x)$, $\lim_{x \to a} h(x) = L$ implies that for every $\epsilon > 0$, there exists $\delta_2 > 0$ such that:

$|x - a| < \delta_2 \implies |h(x) - L| < \epsilon \quad \Rightarrow -\epsilon < h(x) - L < \epsilon \quad (ii)$

Since $g(x) \le f(x) \le h(x)$, subtracting $L$ from all sides gives:

$g(x) - L \le f(x) - L \le h(x) - L$

Let $\delta = \min{\delta_1, \delta_2}$. Then, for $|x - a| < \delta$, we have:

$-\epsilon < g(x) - L \le f(x) - L \le h(x) - L < \epsilon$

This implies:

$-\epsilon < f(x) - L < \epsilon \quad \Rightarrow \lim_{x \to a} f(x) = L$

Thus, the Sandwich Theorem is proved.

Conditions for Applying the Sandwich Theorem

Learn the essential conditions for applying the Sandwich Theorem in calculus, including how to identify bounding functions and verify limits for accurate evaluation of tricky limits.

Function Bounded Between Two Functions

For the Sandwich Theorem (Squeeze Theorem) to be applied, the target function $g(x)$ must be bounded between two functions $f(x)$ and $h(x)$ such that:

$f(x) \le g(x) \le h(x)$

for all $x$ in a neighbourhood of the point $x = a$. This condition ensures that $g(x)$ is “trapped” or “squeezed” between the lower bound $f(x)$ and the upper bound $h(x)$. Recognizing these bounding functions is crucial for solving tricky limits in calculus, especially in NCERT, CBSE, and competitive exam problems.

Limits of Bounding Functions at a Point

The bounding functions $f(x)$ and $h(x)$ must have the same limit at the point of interest $x = a$:

$\lim_{x \to a} f(x) = L = \lim_{x \to a} h(x)$

If this condition is satisfied, the Sandwich Theorem guarantees that:

$\lim_{x \to a} g(x) = L$

This principle is particularly useful for evaluating limits of oscillating functions, where direct substitution may not work.

When the Theorem Cannot Be Applied

The Sandwich Theorem cannot be used if:

• The target function is not properly bounded by two known functions.

• The limits of the bounding functions at the point $x = a$ are different.

• The function is undefined or discontinuous in the neighbourhood of $x = a$.

Understanding these restrictions is important to avoid mistakes while solving limits in exams like JEE, CUET, and other competitive calculus problems.

Step-by-Step Method to Use the Sandwich Theorem

Master a systematic, step-by-step approach to using the Sandwich Theorem, ensuring precise limit calculation for oscillating and complex functions in competitive exams.

Identifying the Bounding Functions

The first step is to identify two functions $f(x)$ and $h(x)$ such that they bound the target function $g(x)$ from below and above:

$f(x) \le g(x) \le h(x)$

Selecting appropriate bounding functions requires analyzing the behavior of $g(x)$ near the point of interest, especially for oscillating or complex functions.

Verifying the Limits of Bounding Functions

Next, calculate the limits of the bounding functions at $x = a$:

$\lim_{x \to a} f(x)$ and $\lim_{x \to a} h(x)$

If both limits are equal to the same value $L$, the function $g(x)$ is “squeezed” and its limit can be determined using the theorem.

Concluding the Limit of the Target Function

Once the bounding functions and their limits are verified, the limit of the target function $g(x)$ can be concluded as:

$\lim_{x \to a} g(x) = L$

This systematic approach ensures accuracy when solving competitive exam calculus problems involving the Squeeze Theorem.

Example 1: $\lim_{x \to 0} x^2 \sin(1/x)$

Here, the function $g(x) = x^2 \sin(1/x)$ is bounded by $-x^2$ and $x^2$:

$-x^2 \le x^2 \sin(1/x) \le x^2$

Since $\lim_{x \to 0} -x^2 = 0 = \lim_{x \to 0} x^2$, by the Sandwich Theorem,

$\lim_{x \to 0} x^2 \sin(1/x) = 0$

This is a classic oscillating function example often asked in JEE and CBSE exams.

Example 2: $\lim_{x \to 0} x \cos(1/x)$

Similarly, $x \cos(1/x)$ is bounded between $-x$ and $x$:

$-x \le x \cos(1/x) \le x$

As $\lim_{x \to 0} -x = 0 = \lim_{x \to 0} x$, it follows that:

$\lim_{x \to 0} x \cos(1/x) = 0$

Example 3: Tricky Oscillating Functions

Other functions like $x \sin(1/x^2)$ or $x^3 \cos(1/x)$ can also be solved using the Squeeze Theorem. The key is always to find appropriate bounding functions and verify their limits.

Sandwich Theorem vs Other Limit Techniques

Understand the differences between the Sandwich Theorem and other limit techniques like L’Hospital’s Rule, and learn when to apply each method for maximum efficiency.

Comparison with L’Hospital’s Rule

Unlike L’Hospital’s Rule, which uses derivatives to solve indeterminate forms like $0/0$ or $\infty/\infty$, the Sandwich Theorem works by bounding a function. It is particularly useful when derivatives are difficult or unnecessary.

When to Use Sandwich Theorem Over Algebraic Methods

The Sandwich Theorem is ideal when:

• The function oscillates or cannot be factored easily.

• The bounding functions and their limits are obvious or simple.

• Algebraic simplification or L’Hospital’s Rule is too complex or inapplicable.

This makes the Squeeze Theorem a preferred method for NCERT, JEE, CUET, and competitive exam problems.

Solved Examples Based On Sandwich Theorem:

Example 1: For each $\mathrm{t} \in \mathrm{R}$, let $[\mathrm{t}]$ be the greatest integer less than or equal to $t$. Then $\lim _{x \rightarrow 0} x\left(\left[\frac{1}{x}\right]+\left[\frac{2}{x}\right]+\ldots \ldots+\left[\frac{15}{x}\right]\right)$
1) does not exist (in $R$).
2) is equal to $0$.
3) is equal to $15$
4) is equal to $120$

Solution:

The SANDWICH THEOREM -
If $f(x) \leq g(x) \leq h(x)$ for every $(x)$ in the deleted neighbourhoodo $f(a)$.
and $\lim _{x \rightarrow a} f(x)=\lim _{x \rightarrow a} h(x)=l$

Then $\lim _{x \rightarrow a} g(x)$ is also equal to $l$
- wherein

Where $x \epsilon(a-\delta, a+\delta)$ and $\delta$ is very small.

$
\begin{aligned}
& L=\lim _{x \rightarrow 0^{+}} x\left[\left(\frac{1}{x}\right)+\ldots+\left(\frac{15}{x}\right)\right] \\
& L=\lim _{x \rightarrow 0^{+}} x\left(\frac{1}{x}\right)+x\left(\frac{2}{x}\right)+\ldots+x\left(\frac{15}{x}\right)
\end{aligned}
$

Take $\lim _{x \rightarrow 0+} x\left[\frac{1}{x}\right]$
Let $\frac{1}{x}=y$
when $\mathrm{x} \rightarrow 0, \mathrm{y} \rightarrow \infty$

$
\mathrm{L}=\lim _{y \rightarrow \infty} \frac{1}{y} \mathrm{y}
$

Let $\mathrm{y}=\mathrm{n}+\mathrm{p}$, when $\mathrm{n} \rightarrow \infty \quad, 0<\mathrm{p}<1$

$
\begin{aligned}
& \lim _{n \rightarrow \infty} \frac{n}{n+p} \\
& \lim _{n \rightarrow \infty} \frac{1}{1+\frac{p}{n}}=1
\end{aligned}
$

Take $\lim _{x \rightarrow 0^{+}} x\left[\frac{2}{x}\right]$
Let $\frac{2}{x}=y$
when $x \rightarrow 0, y \rightarrow \infty$

$
\begin{aligned}
& \lim _{y \rightarrow \infty} \frac{2}{y} y=2 \\
& \lim _{x \rightarrow 0+} x\left[\frac{1}{x}\right]+x\left[\frac{2}{x}\right]+----+x\left[\frac{15}{x}\right] \\
& =1+2+3+--\cdots+15 \\
& =\frac{n(n+1)}{2} \\
& =\frac{15 \times 16}{2} \\
& = 120
\end{aligned}
$

Example 2: Which of the following is true for the real valued functions $f(x), h(x), g(x)$

$
\lim _{x \rightarrow a} f(x)=L, \lim _{x \rightarrow-a} g(x)=L, \lim _{x \rightarrow-a} f(x)=N, \lim _{x \rightarrow a} g(x)=N \text { ? }
$

a) Sandwich theorems can be applied to find $\lim _{x \rightarrow a} h(x) \lim _{x \rightarrow a} f(x)$ and $\lim _{x \rightarrow a} g(x)$ exists.
b) Sandwich theorems can be applied to find $\lim _{x \rightarrow a} h(x) \lim _{x \rightarrow a} f(x)$ and $\lim _{x \rightarrow a} h(x)$ exists.
c) Both a and b
d) None of the above

Solution:

Note the following points.
- The limit of any function $\lim _{x \rightarrow p} f(x)$ may exist even when $\mathrm{f}(\mathrm{x})$ is not defined at $x=p$
- The application of the Sandwich theorem needs the real-valued functions to share the same domain of consideration.
- The application of the Sandwich theorem needs the limits $\lim _{x \rightarrow a} f(x)$ and $\lim _{x \rightarrow a} g(x)$ or $\lim _{x \rightarrow a} f(x)$ and $\lim _{x \rightarrow a} g(x)$.
- It is true from the following that the limits limits $\lim _{x \rightarrow a} f(x)$ and $\lim _{x \rightarrow a} g(x)$ or $\lim _{x \rightarrow a} f(x)$ and $\lim _{x \rightarrow a} g(x)$ exists.

But the domains of the real-valued functions $f(x), h(x), g(x)$
must be defined and the same for the application of the Sandwich theorem in the said limits.
Hence, the answer is option (4).

Example 3: Find this limit with the help of the squeeze theorem $\lim _{x \rightarrow 0}\left[x^2 \sin (\pi x)\right], \quad \forall x \in R$.
1) $\pm \pi$
2) $1$
3) $0$
4) $\pi$

Solution:

The Sandwich theorem also known as the squeeze play theorem applicable for any three real-valued functions $f(x), g(x)$ and $h(x)$ that share the same domain such that $f(\mathrm{x}) \leq g(\mathrm{x}) \leq h(\mathrm{x})$ for $\nabla x$ in the domain of definition states the following:
For some real value of $\alpha$ if $\lim _{x \rightarrow a} f(x)=l=\lim _{x \rightarrow a} h(x)$, then $\lim _{x \rightarrow a} f(x)=l$
Note that for $\forall x \in R$, the range of the sine function is

$
-1 \leq \sin (\pi x) \leq 1-(1)
$

Now, multiply the inequality (i) by the square of that still preserves the inequality.

$
\begin{aligned}
& \left(x^2\right) \times(-1) \leq\left(x^2\right) \times \sin (\pi x) \leq\left(x^2\right) \times 1 \\
& -x^2 \leq x^2 \sin (\pi x) \leq x^2 \\
& -x^2 \leq x^2 \sin (\pi x) \leq x^2 \quad \ldots \text { (ii) }
\end{aligned}
$

Apply the squeeze theorem to the inequality (ii).

$
\begin{aligned}
& \lim _{x \rightarrow 0}\left(-x^2\right) \leq \lim _{x \rightarrow 0}\left(x^2 \sin (\pi x)\right) \leq \lim _{x \rightarrow 0} x^2 \\
& \left(\lim _{x \rightarrow 0}(-x)\right)^2 \leq \lim _{x \rightarrow 0}\left(x^2 \sin (\pi x)\right) \leq\left(\lim _{x \rightarrow 0} x\right)^2 \\
& 0 \leq \lim _{x \rightarrow 0}\left(x^2 \sin (\pi x)\right) \leq 0
\end{aligned}
$

Therefore, the required value of the limit is

$
\lim _{x \rightarrow 0}\left(x^2 \sin (\pi x)\right)=0
$

Hence, the answer is option (3).

Example 4: The value of $\lim\limits _{n \rightarrow \infty} \frac{[r]+[2 r]+\ldots+[n r]}{n^2}$where r is a non-zero real number and $[r]$ denoted the greatest integer less than or equal to r, is equal to : [JEE MAIN 2021]

1) $\frac{r}{2}$
2) $r$
3) $2 r$
4) 0

Solution:

As we know that

$
\begin{gathered}
\mathrm{r}-1<[\mathrm{r}] \leq \mathrm{r} \\
2 \mathrm{r}-1<[2 \mathrm{r}] \leq 2 \mathrm{r} \\
\vdots \\
\mathrm{nr}-1<[\mathrm{nr}] \leq \mathrm{nr}
\end{gathered}
$
Add all this

$
\begin{aligned}
& (\mathrm{r}+2 \mathrm{r}+\ldots \ldots+\mathrm{nr})-\mathrm{n}<[\mathrm{r}]+[2 \mathrm{r}]+\ldots \ldots+[\mathrm{nr}] \leq \mathrm{r}+2 \mathrm{r}+\ldots \ldots+\mathrm{nr}_{\text {Now }} \\
& \frac{(\mathrm{r}+2 \mathrm{r}+\ldots .+\mathrm{nr})-\mathrm{n}}{\mathrm{n}^2}<\frac{[\mathrm{r}]+[2 \mathrm{r}]+\ldots \ldots+[\mathrm{nr}]}{\mathrm{n}^2} \leq \frac{\mathrm{r}+2 \mathrm{r}+\ldots \ldots+\mathrm{nr}}{\mathrm{n}^2} \\
& \frac{\frac{n(n+1)}{2} \cdot r-n}{n^2}<\frac{[r]+[2 r]+\ldots .+[n r]}{n^2} \leq \frac{\frac{n(n+1)}{2} r}{n^2}
\end{aligned}
$
Now,
$\lim\limits _{n \rightarrow \infty} \frac{\frac{n(n+1)}{2} \cdot r-n}{n^2}=\frac{r}{2}$
$\lim\limits _{n \rightarrow \infty} \frac{\frac{n(n+1) r}{2}}{n^2}=\frac{r}{2}$
By sandwich theorem

$
\lim\limits _{n \rightarrow \infty} \frac{[r]+[2 r]+\ldots \ldots+[n r]}{n^2}=\frac{r}{2}
$
Hence, the answer is the option 1.

Example $5: \lim\limits _{n \rightarrow \infty}\left\{\left(2^{\frac{1}{2}}-2^{\frac{1}{3}}\right)\left(2^{\frac{1}{2}}-2^{\frac{1}{5}}\right) \ldots\left(2^{\frac{1}{2}}-2^{\frac{1}{22 n+1}}\right)\right\} {\text { is equal to: }}$
[JEE MAIN 2023]
1) $\frac{1}{\sqrt{2}}$
2) $\sqrt{2}$
3) 1
4) 0

Solution:
$
\begin{aligned}
& P=\lim\limits _{n \rightarrow \infty}\left(2^{\frac{1}{2}}-2^{\frac{1}{3}}\right)\left(2^{\frac{1}{2}}-2^{\frac{1}{5}}\right) \ldots \cdots\left(2^{\frac{1}{2}}-2^{\frac{1}{2 n+1}}\right) \\
& \text { Let } \\
& 2^{\frac{1}{2}}-2^{\frac{1}{3}} \quad \rightarrow \text { Smallest } \\
& 2^{\frac{1}{2}}-2^{\frac{1}{2 n+1}} \rightarrow \text { Largest }
\end{aligned}
$
Using Sandwich theorem:

$
\begin{aligned}
& \left(2^{\frac{1}{2}}-2^{\frac{1}{3}}\right)^n \leq P \leq\left(2^{\frac{1}{2}}-2^{\frac{1}{2 n+1}}\right)^n \\
& \binom{\operatorname{lie}^n b / w}{0 \text { and } 1}^n \\
& \lim\limits _{n \rightarrow \infty}\left(2^{\frac{1}{2}}-2^{\frac{1}{3}}\right)^n=0 \\
& \lim\limits _{n \rightarrow \infty}\left(2^{\frac{1}{2}}-2^{\frac{1}{2 n+1}}\right)^n=0 \\
& \therefore \mathrm{P}=0
\end{aligned}
$

Hence, the answer is the option 4.

List of topics related to Limits

Check out a comprehensive list of topics related to limits, including indeterminate forms, Squeeze Theorem, and continuity, essential for CBSE, JEE, and CUET preparation.

Indeterminate forms of limits

Limits of Trigonometric Functions

Limits using expansion

Left and Right Hand Limits

Higher Order Derivatives

NCERT Resources

Access NCERT-aligned resources, notes, and exemplar solutions for the chapter 13 Limits and Derivatives to strengthen your understanding of the Sandwich Theorem and other limit concepts in calculus.

NCERT Notes for Class 11 Maths Chapter 13 - Limits and Derivatives

NCERT Solutions for Class 11 Maths Chapter 13 - Limits and Derivatives

NCERT Exempar Solutions for Class 11 Maths Chapter 13 - Limits and Derivatives

Practice Questions based on Sandwich Theorem

Practice well-structured questions on the Sandwich Theorem, ranging from basic NCERT problems to advanced competitive exam questions for better mastery of limits.

Sandwich Theorem - Practice Question MCQ

We have shared the links below to practice questions on the related topics of limits: