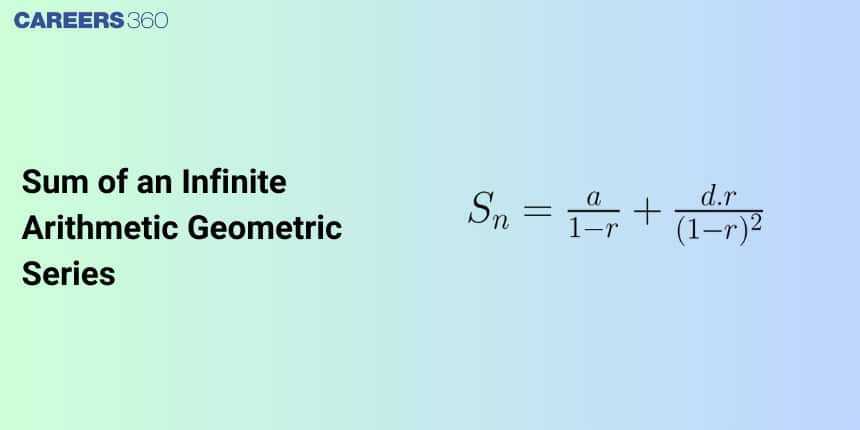
Sum of an Infinite Arithmetic Geometric Series

Arithmetico- Geometric series is a series with a combination of the Arithmetic series and Geometric series. This series is formed by taking the product of the corresponding elements of arithmetic and geometric progressions. In short form, it is written as A.G.P (Arithmetico-Geometric Progression). In real life, we use the sum of an Infinite Arithmetic Geometric Series for analyzing current flow and sound waves.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas  | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics 

This Story also Contains

1. Arithmetico-Geometric Series
2. The sum of an Infinite Arithmetico Geometric Series
3. Summary
4. Solved Examples Based on Arithmetico-Geometric Series

In this article, we will cover the concept of the Sum of an Infinite Arithmetic Geometric Series. This category falls under the broader category of Sequence and series, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Questions based on this topic have been asked frequently in JEE Mains.

Arithmetico-Geometric Series

Arithmetico-geometric series is the combination of arithmetic and geometric series. This series is formed by taking the product of the corresponding elements of arithmetic and geometric progressions. In short form, it is written as A.G.P (Arithmetico-Geometric Progression).

Let the given AP be $a,(a+d),(a+2 d),(a+3 d)$, $\qquad$
And, the GP is $1, r, r^2, r^3, \ldots \ldots$
Multiplying the corresponding elements of the above progression, we get, $a,(a+d) r,(a+2 d) r^2,(a+3 d) r^3, \ldots \ldots$
This is a standard Arithmetico-Geometric Progression.
Eg: $1,3 x, 5 x^2, 7 x^3, 9 x^4, \ldots \ldots$

The sum of an Infinite Arithmetico Geometric Series

$\mathrm{S}_{\infty}$ denotes the sum of an infinite AGP. This sum is a finite quantity if -1 < r < 1

$
\mathrm{S}_{\infty}=a+(a+d) r+(a+2 d) r^2+(a+3 d) r^3 \ldots \ldots
$

Multiply both side of eq (i) by 'r '
$
r \mathrm{~S}_{\infty}=a r+(a+d) r^2+(a+2 d) r^3+(a+3 d) r^4 \ldots \ldots .
$

Subtract eq (ii) from eq (i)
$
\begin{aligned}
& (1-r) \mathrm{S}_{\infty}=a+\left(d r+d r^2+d r^3+\ldots . . \text { upto } \infty\right) \\
& \Rightarrow(1-r) \mathrm{S}_{\infty}=a+\frac{d r}{1-r} \\
& \Rightarrow \mathbf{S}_{\infty}=\frac{\mathbf{a}}{\mathbf{1 - \mathbf { r }}}+\frac{\mathbf{d r}}{(\mathbf{1 - r})^2}
\end{aligned}
$

The sum of infinite AGP is given by
$
S_{\infty}=\frac{a}{1-r}+\frac{d r}{(1-r)^2}
$
here $|r|<1$

Where,

a= first term of AGP

d= common difference of AP

r= Common ratio of GP

Summary

Arithmetic-geometric series are the linear and exponential growth patterns of arithmetic and geometric progressions. Arithmetico - Geometric series has wide applications in the fields of mathematics, physics, and astrology. Understanding of Arithmetic- Geometric progressions help us to analyze and solve complex real-life problems.

Recommended Video :

Solved Examples Based on Arithmetico-Geometric Series

Example 1: If the sum of the series $
\left(\frac{1}{2}-\frac{1}{3}\right)+\left(\frac{1}{2^2}-\frac{1}{2 \cdot 3}+\frac{1}{3^2}\right)+\left(\frac{1}{2^3}-\frac{1}{2^2 \cdot 3}+\frac{1}{23^2}-\frac{1}{3^5}\right)+\left(\frac{1}{2^4}-\frac{1}{2^{23}}+\frac{1}{2^{2 \cdot 3^2}}-\frac{1}{2^2 \cdot 3^2}+\frac{1}{3^4}\right)+
$ is $\frac{\alpha}{\beta}$
, where $\alpha$ and $\beta$ are co-prime, then $\alpha+3 \beta$ is equal to [JEE MAINS 2023]

Solution: The sum of the series represents Arithmetico - Geometric Progression,
$
\begin{aligned}
& P=\left(\frac{1}{2}-\frac{1}{3}\right)+\left(\frac{1}{2^2}-\frac{1}{2 \cdot 3}+\frac{1}{3^2}\right)+\left(\frac{1}{2^3}+\frac{1}{2^2 \cdot 3}+\frac{1}{2 \cdot 3^2}-\frac{1}{3^2}\right)+\ldots P\left(\frac{1}{2}+\frac{1}{3}\right)=\left(\frac{1}{2^2}-\frac{1}{3^2}\right)+\left(\frac{1}{2^3}+\frac{1}{3^3}\right)+\left(\frac{1}{2^4}-\frac{1}{3^4}\right)+\ldots \\
& \frac{5 P}{6}=\frac{\frac{1}{6}}{1-\frac{1}{2}}-\frac{\frac{1}{8}}{1+\frac{1}{3}} \\
& \frac{5 P}{6}=\frac{1}{2}-\frac{1}{12}-\frac{5}{12} \\
& \therefore P=\frac{1}{2}=\frac{\alpha}{\beta} \quad \therefore \alpha=1, \beta=2 \\
& \alpha+3 B=7
\end{aligned}
$

Hence, the required answer is 7.

Example 2: Suppose $\mathrm{a}_1, \mathrm{a}_2, 2, \mathrm{a}_3, \mathrm{a}_4$ be in an arithemetico-geometric progression. If the common ratio of the corresponding geometric progression is 2 and the sum of all 5 terms of the arithmetico-geometri 49 progression is $\frac{49}{2}$, then $a_4$ is equal to $\qquad$ [JEE MAINS 2023]

Solution:
Given,
Common ratio $=\mathrm{r}=2$

The sum of all 5 terms of the arithmetico- geometric progression is $\frac{49}{2}$
$
\begin{aligned}
& \frac{(a-2 d)}{4}, \frac{(a-d)}{2}, a, 2(a+d), 4(a+2 d) \\
& a=2 \\
& \left(\frac{1}{4}+\frac{1}{2}+1+6\right) \times 2+(-1+2+8) d=\frac{49}{2} \\
& 2\left(\frac{3}{4}+7\right)+9 d=\frac{49}{2} \\
& 9 d=\frac{49}{2}-\frac{62}{4}=\frac{98-62}{4}=9 \\
& d=1 \\
& \Rightarrow a_4=4(a+2 d) \\
& =16
\end{aligned}
$

Hence, the required answer is 16.

Example 3: Let $a_1, a_2, a_3, \ldots$ be an A.P. If $\sum_{r=1}^{\infty} \frac{a_r}{2^r}=4$, then $4 a_2$
is equal to $\qquad$ [JEE MAINS 2022]
Solution
$
\begin{aligned}
& \mathrm{b}=\mathrm{s}=\frac{\mathrm{a}_1}{2}+\frac{\mathrm{a}_2}{2^2}+\frac{\mathrm{a}_3}{2^3}+\cdots \\
& \frac{\mathrm{s}}{2}=\frac{\mathrm{a}_4}{2^2}+\frac{\mathrm{a}_2}{2^3}+\cdots \\
& \frac{\mathrm{s}}{2}=\frac{\mathrm{a}_1}{2}+\mathrm{d}\left(\frac{1}{2^2}+\frac{1}{2^3}+\cdots\right. \\
& \frac{\mathrm{s}}{2}=\frac{\mathrm{a}_1}{2}+\mathrm{d}\left(\frac{\frac{1}{4}}{1-\frac{1}{2}}\right) \\
& \therefore \mathrm{s}=\mathrm{a}_1+\mathrm{d}_1=\mathrm{a}_2=4 \\
& \text { or } 4 \mathrm{a}_2=16
\end{aligned}
$

Hence, the required answer is 16.

Example 4: If $\frac{6}{3^{12}}+\frac{10}{3^{11}}+\frac{20}{3^{10}}+\frac{40}{3^9}+\ldots+\frac{10240}{3}=2^{\mathrm{n}} \cdot \mathrm{m}$, where m is odd, then $\mathrm{m} . \mathrm{n}$ is equal to $\qquad$ [JEE MAINS 2022]
Solution: The given series represents Arithmetico- Geometric Progression,
$
\begin{aligned}
& \frac{6}{3^{12}}+\frac{10}{3^{11}}+\frac{20}{3^{10}}+\cdots+\frac{10240}{3^1} \\
& =\frac{1}{3^{12}}+\left(\frac{5}{3^{12}}+\frac{10}{3^{11}}+\cdots+\frac{10240}{3^1}\right)
\end{aligned}
$
G.p. with $\mathrm{r}=2 \times 3=6, \mathrm{n}=12$
$
\begin{aligned}
& =\frac{1}{3^{12}}+\frac{5}{3^{12}}\left(\frac{6^{12}-1}{6-1}\right) \\
& =\frac{1}{3^{12}}+\frac{6^{12}-1}{3^{12}} \\
& =\frac{6^{12}}{3^{12}} \\
& =2^{12} \\
& \therefore m=1, n=12 \\
& \therefore m \cdot n=12
\end{aligned}
$

Hence, the answer is the 12.

Example 5: Let $\mathrm{S}=2+\frac{6}{7}+\frac{12}{7^2}+\frac{20}{7^3}+\frac{30}{7^4}+\ldots$ Then 4 S is equal to
Solution: The given series represents Arithmetico-Geometric Progression, [JEE MAINS 2022]
$
\begin{aligned}
& \mathrm{S}=2+\frac{6}{7}+\frac{12}{7^2}+\frac{20}{7^3}+\frac{30}{7^4}+\cdots \\
& \frac{S}{7}=\frac{2}{7}+\frac{6}{7^2}+\frac{12}{7^3}+\frac{20}{7^4}+\cdots \\
& \text { Subtract } \quad \frac{6 \mathrm{~S}}{7}=2+\frac{4}{7}+\frac{6}{7^2}+\frac{8}{7^3}+\frac{10}{7^4}+\cdots \\
& \Rightarrow \quad \frac{6}{7^2} \mathrm{~S}=\frac{2}{7}+\frac{4}{7^2}+\frac{6}{7^3}+\frac{8}{7^4}+\cdots \\
&
\end{aligned}
$

\begin{aligned}
& \text { Subtract } \Rightarrow \frac{6}{7}\left(1-\frac{1}{7}\right) S=2+\frac{2}{7}+\frac{2}{7^2}+\frac{2}{7^3}+\frac{2}{7^4}+\cdots \\
& \Rightarrow \frac{36}{49} \mathrm{~S}=2\left(\frac{1}{1-1 / 7}\right) \\
& \Rightarrow \frac{36}{49} \mathrm{~S}=\frac{7}{3} \Rightarrow \mathrm{S}=\frac{7^3}{2^2 \cdot 3^3} \\
& \Rightarrow 4 \mathrm{~S}=\left(\frac{7}{3}\right)^3 \\
&
\end{aligned}

Hence, the required answer is $\left(\frac{7}{3}\right)^3$