Summation Formula (With Solved Example)

Q: How do you represent the sum of the n term of AP by sigma notation?

The sum of n terms of A.P. whose rth term is = a+ (r-1)*d; where a is the first term and d is the common difference is given by

SUMMATION FORMULA

Edited By Komal Miglani | Updated on Sep 18, 2024 06:03 PM IST

Summation is the addition of a sequence of numbers, called addends or summands; the result is their sum or total. If we add or subtract all the terms of a sequence we will get an expression, which is called a series. A series can be simply represented using summation, often known as sigma notation. In real life, we use Summation in mathematics and statistics to represent the sum of a series of numbers.

JEE Main 2025: Sample Papers | Mock Tests | PYQs | Study Plan 100 Days

JEE Main 2025: Maths Formulas | Study Materials

JEE Main 2025: Syllabus | Preparation Guide | High Scoring Topics

This Story also Contains

Summation by Sigma(Σ) Operator
Properties of Sigma Notation
Solved Examples Based on Summation by Sigma Operator

In this article, we will cover the concept of Summation by Sigma Operator. This category falls under the broader category of Sequence and series, which is a crucial Chapter in class 11 Mathematics. It is not only essential for board exams but also for competitive exams like the Joint Entrance Examination(JEE Main) and other entrance exams such as SRMJEE, BITSAT, WBJEE, BCECE, and more. Over the last ten years of the JEE Main Exam (from 2013 to 2023), a total of 20 questions have been asked on this concept, including one in 2029, four in 2021, two in 2022, and twelve in 2023.

Summation by Sigma(Σ) Operator

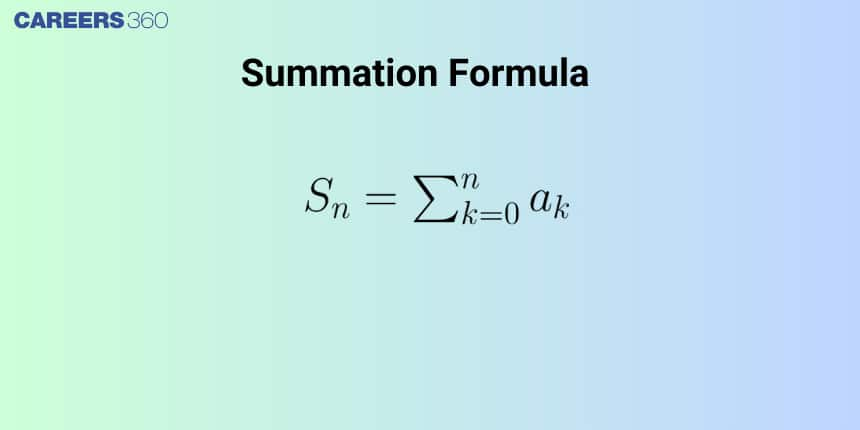
The summation of each term of a sequence or a series can be represented in a compact form, called summation or sigma notation. This summation is represented by the Greek capital letter, Sigma (Σ).

For example,

$\\\mathrm{\sum_{n=1}^{n=10} n\;,\;it\;means \;the \;sum\;of\;n\;terms\;when\;n\;varies\;from\;1\;to\;10}\\\mathrm{\sum_{n=1}^{n=10} n=1+2+3+4+5+6+7+8+9+10}$

If we have the formula for the rth term i.e. $A_r$ of the series, we can put the sum of n terms of the series in the form of sigma notation as

$S_{n}= a_{1}+ a_{2}+--------+ a_{n}$ = $\sum^n_{r=1} A_r$

Here, $A_r$ is called the general term of the series.

Thus, the sum of n terms of A.P. whose rth term is $A_r$ = a+ (r-1)*d; where a is the first term and d is the common difference is given by

$S_n = \sum^n_{r=1} A_r =\sum ^n_{r=1} a+ (r-1)*d$

In fact, we can put the sum of any series in the sigma notation if the formula for its rth term is known.

Properties of Sigma Notation

$\\\mathrm{1.\;\;\sum_{r=1}^{n}T_r=T_1+T_2+T_3+.......+T_n,\;where,\;T_r\;is\;the\;general\;term\;of\;the\;series.}\\\\\mathrm{2.\;\;\sum_{r=1}^{n}\left ( T_r\pm T_r' \right )=\sum_{r=1}^{n}T_r\pm\sum_{r=1}^{n}T_r'\;\;(sigma\;\;operator\;is\;distributive\;\;over\;addition\;and\;subtraction)}\\\\\mathrm{3.\;\;\sum_{r=1}^{n}T_rT_r'\neq\left ( \sum_{r=1}^{n}T_r \right )\left ( \sum_{r=1}^{n}T_r' \right )\;\;(sigma\;\;operator\;is\;not\;distributive\;\;over\;multiplication)}\\\\\mathrm{4.\;\;\sum_{r=1}^{n}\frac{T_r}{T_r'}\;\neq\;\frac{\sum_{r=1}^{n}T_r}{\sum_{r=1}^{n}T_r'}\;\;(sigma\;\;operator\;is\;not\;distributive\;\;over\;division})\\\\\mathrm{5.\;\;\sum_{r=1}^{n}aT_r=a\sum_{r=1}^{n}T_r\;\;\;\;(a\;is\;constant)}\\\\\mathrm{6.\;\;\sum_{j=1}^{n}\sum_{i=1}^{n}T_iT_j=\left ( \sum_{i=1}^{n}T_i \right )\left ( \sum_{j=1}^{n}T_j \right )\;\;\;(here\;i\;and\;j\;are\;independent)}$

Solved Examples Based on Summation by Sigma Operator

Example 1: Let $<a_n>$ be a sequence such that $a_1+a_2+\ldots+a_a=\frac{n^2+3 n}{(n+1)(n+2)}$ , If $28 \sum_{k=1}^{10} \frac{1}{a_k}=p_1 p_2 p_3 \ldots p_m$ , where $p_1, p_2 \ldots \ldots \mathrm{P}_w$ are the first m prime numbers, then m is equal to [JEE MAINS 2023]

Solution

$\begin{aligned} & a_n=S_n-S_{n-1}=\frac{n^2+3 n}{(n+1)(1+2)}-\frac{(n-1)(n+2)}{n(n+1)} \\ & \Rightarrow a_n=\frac{4}{n(n+1)(1+2)} \\ & \Rightarrow 28 \sum_{k-1}^{10} \frac{1}{a_k}=28 \sum_{k=1}^{10} \frac{k(k+1)(k+2)}{4} \\ & =\frac{7}{4} \sum_{k=1}^{10}(k(k+1)(k+2)(k+3)-(k-1) k(k+1)(k+2) \\ & =\frac{7}{4} .10 .11 .12 .13=2.3 .5 .7 .11 .13 \\ & \text { So } m=6 \\ & \end{aligned}$

Hence, the answer is 6

Example 2: Let $\sum_{n=0}^{\infty} \frac{n^3((2 n) !)+(2 n-1)(n !)}{(n !)((2 n) !)}=a e+\frac{b}{e}+c$ , where $\text { a, b, c } \in \mathbb{Z}$ and $\mathrm{e}=\sum_{n=0}^{\infty} \frac{1}{n !}$ Then $a^2-b+c$ is equal to : [JEE MAINS 2023]

Solution

$\begin{aligned} & \text { Let } \sum_{n=0}^{\infty} \frac{n^3((2 n) !)+(2 n-1) n !}{(n !)((2 n) !)} \\ & =\sum_{n=0}^{\infty} \frac{n^3(2 n) !}{n !(2 n) !}+\frac{(2 n-1) n !}{n !(2 n) !} \\ & =S_1+S_2 \end{aligned}$

$\text { Let } \begin{aligned} S_1 & =\sum_{n=0}^{\infty} \frac{n^3(2 n) !}{n !(2 n) !}=\sum_{n=0}^{\infty} \frac{n^3}{n !}=\sum_{n=1}^{\infty} \frac{n^2}{(n-1) !} \\ & =\sum_{n=1}^{\infty} \frac{n^2-1+1}{(n-1) !} \\ & =\sum_{n=2}^{\infty} \frac{(n+1)}{(n-2) !}+\sum_{n=1}^{\infty} \frac{1}{(n-1) !} \end{aligned}$

$\begin{aligned} & =\sum_{n=2}^{\infty} \frac{(n-2)+3}{(n-2) !}+\sum_{n=1}^{\infty} \frac{1}{(n-1) !} \\ & =\sum_{n=3}^{\infty} \frac{1}{(n-3) !}+3 \sum_{n=2}^{\infty} \frac{1}{(n-2) !}+\sum_{n=1}^{\infty} \frac{1}{(n-1) !} \\ & S_1=e+3 e+e=5 e \\ & \because S_2=\sum_{n=0}^{\infty} \frac{(2 n-1) n !}{n !(2 n) !} \\ & =\sum_{n=0}^{\infty} \frac{2 n-1}{(2 n) !} \end{aligned}$

$\begin{aligned} & =\sum_{n=1}^{\infty} \frac{1}{(2 n-1) !}-\sum_{n=0}^{\infty} \frac{1}{(2 n) !} \\ & =\left(\frac{1}{1 !}+\frac{1}{3 !}+\frac{1}{5 !}+\ldots\right)-\left(1+\frac{1}{2 !}+\frac{1}{4 !}+\ldots .\right) \\ & =-1+\frac{1}{1 !}-\frac{1}{2 !}+\frac{1}{3 !}-\frac{1}{4 !}+\frac{1}{5 !}-\ldots \\ & =-\left(1-\frac{1}{1 !}+\frac{1}{2 !}-\frac{1}{3 !}+\frac{1}{4 !}+\ldots .\right) \\ & =-\mathrm{e}^{-1} \end{aligned}$

$\mathrm{S}_1+\mathrm{S}_2=5 \mathrm{e}-\frac{1}{\mathrm{e}}=\mathrm{ae}+\frac{\mathrm{b}}{\mathrm{e}}+\mathrm{c}$

Compare both sides

$\begin{aligned} & \mathrm{a}=5, \mathrm{~b}=-1, \mathrm{c}=0 \\ & \mathrm{a}^2-\mathrm{b}+\mathrm{c}=25+1+0=26 \end{aligned}$

Hence, the answer is 26.

Example 3: Let f(x) be a function such that $f(x+y)=f(x) \cdot f(y)$ for all $x, y \in N$ if $f(1)=3$ and $\sum_{\mathrm{k}=1}^{\mathrm{n}} \mathrm{f}(\mathrm{k})=3279$ then the value of n is. [JEE MAINS 2023]

Solution

$\begin{aligned} &\begin{aligned} & f(x+y)=f(x) \cdot f(y), x, y \in N \\ & f(2)=3^2 \end{aligned}\\ &\begin{aligned} f(3)=3^3 \quad & \therefore 3 \frac{\left[3^n-1\right]}{2}=3279 \\ & 3^n-1=1093 \times 2 \\ & 3^n-1=2186 \\ & 3^n=2187 \\ & n=7 \end{aligned} \end{aligned}$

Hence, the answer is 7

Example 4: Let $\mathrm{s}_1, \mathrm{~s}_2, \mathrm{~s}_3, \ldots \ldots, \mathrm{s}_{10}$ respectively be the sum to 12 terms of 10 A.P. s whose first terms are $1,2,3, \ldots, 10$ and the common differences are $1,3,5, \ldots \ldots \ldots, 19$ respectively. Then $\sum_{\mathrm{i}=1}^{10} \mathrm{~s}_{\mathrm{i}}$ is equal to [JEE MAINS 2023]

Solution

$\begin{aligned} & \mathrm{S}_{\mathrm{k}}=6(2 \mathrm{k}+(11)(2 \mathrm{k}-1)) \\ & \mathrm{S}_{\mathrm{k}}=6(2 \mathrm{k}+22 \mathrm{k}-11) \\ & \mathrm{S}_{\mathrm{k}}=144 \mathrm{k}-66 \\ \end{aligned}$

$\begin{aligned} & \sum_1^{10} \mathrm{~S}_{\mathrm{k}}=144 \sum_{\mathrm{k}=1}^{10} \mathrm{k}-66 \times 10 \\ & =144 \times \frac{10 \times 11}{2}-660 \\ & =7920-660 \\ & =7260 \end{aligned}$

Hence, the answer is 7260

Example 5: Let $[\alpha]$ denote the greatest integer $\leq \alpha$ . Then $[\sqrt{1}]+[\sqrt{2}]+[\sqrt{3}]+\ldots .+[\sqrt{120}]$ is equal to _________. [JEE MAINS 2023]

Solution

$\begin{aligned} & \mathrm{S}=[\sqrt{1}]+[\sqrt{2}]+[\sqrt{3}]+\ldots+[\sqrt{120}] \\ & {[\sqrt{1}] \rightarrow[\sqrt{3}]=1 \times 3} \\ & {[\sqrt{4}] \rightarrow[\sqrt{8}]=2 \times 5} \\ & {[\sqrt{9}] \rightarrow[\sqrt{15}]=3 \times 7} \\ & \vdots \\ & {[\sqrt{100}] \rightarrow[\sqrt{120}]=10 \times 21} \\ & \mathrm{~S}=1 \times 3+2 \times 5+3 \times 7+\ldots+10 \times 21 \\ & =\sum_{\mathrm{r}=1}^{10} \mathrm{r}(2 \mathrm{r}+1) \\ & =2 \sum_{\mathrm{r}=1}^{10} \mathrm{r}^2+\sum_{\mathrm{r}=1}^{10} \mathrm{r} \\ & =\frac{2 \times 10 \times 11 \times 21}{6}+\frac{10 \times 11}{2} \\ & =770+55 \\ & =825 \end{aligned}$

Hence, the answer is (825).

Frequently Asked Questions (FAQs)

1. What is summation?

The summation of each term of a sequence or a series can be represented in a compact form, called summation or sigma notation. This summation is represented by the Greek capital letter, Sigma (Σ).

2. How do you represent the sum of the n term of AP by sigma notation?

The sum of n terms of A.P. whose rth term is $A_r$ = a+ (r-1)*d; where a is the first term and d is the common difference is given by

$S_n = \sum^n_{r=1} A_r =\sum ^n_{r=1} a+ (r-1)*d$